Advertisements
Advertisements
प्रश्न
State whether the following are true or false. Justify your answer.
sin θ = `4/3`, for some angle θ.
पर्याय
True
False
उत्तर
This statement is False.
Explanation:
sin θ = `4/3`
We know that in a right-angled triangle,
sin θ = `("Side opposite to ∠θ")/"Hypotenuse"`
In a right-angled triangle, hypotenuse is always greater than the remaining two sides. Therefore, such value of sin θ is not possible.
Hence, the given statement is false.
संबंधित प्रश्न
If sin A = `3/4`, calculate cos A and tan A.
If ∠A and ∠B are acute angles such that cos A = cos B, then show that ∠A = ∠B.
In ΔABC, right angled at B. If tan A = `1/sqrt3` , find the value of
- sin A cos C + cos A sin C
- cos A cos C − sin A sin C
State whether the following are true or false. Justify your answer.
sec A = `12/5` for some value of angle A.
In the following, trigonometric ratios are given. Find the values of the other trigonometric ratios.
`sec theta = 13/5`
If 3 tan θ = 4, find the value of `(4cos theta - sin theta)/(2cos theta + sin theta)`
if `sec A = 17/8` verify that `(3 - 4sin^2A)/(4 cos^2 A - 3) = (3 - tan^2 A)/(1 - 3 tan^2 A)`
Evaluate the following
`2 sin^2 30^2 - 3 cos^2 45^2 + tan^2 60^@`
Evaluate the Following
`(sin 30^@ - sin 90^2 + 2 cos 0^@)/(tan 30^@ tan 60^@)`
Evaluate the Following
`4/(cot^2 30^@) + 1/(sin^2 60^@) - cos^2 45^@`
In ΔABC is a right triangle such that ∠C = 90° ∠A = 45°, BC = 7 units find ∠B, AB and AC
If sin A = `1/2`, then the value of cot A is ______.
Given that sinα = `1/2` and cosβ = `1/2`, then the value of (α + β) is ______.
Prove the following:
If tan A = `3/4`, then sinA cosA = `12/25`
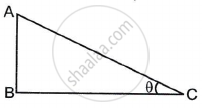
In ΔABC, ∠ABC = 90° and ∠ACB = θ. Then write the ratios of sin θ and tan θ from the figure.
Find the value of sin 45° + cos 45° + tan 45°.
What will be the value of sin 45° + `1/sqrt(2)`?
If sinθ = `1/sqrt(2)` and `π/2 < θ < π`. Then the value of `(sinθ + cosθ)/tanθ` is ______.
If cosec θ = `("p" + "q")/("p" - "q")` (p ≠ q ≠ 0), then `|cot(π/4 + θ/2)|` is equal to ______.
