Advertisements
Advertisements
प्रश्न
In ΔABC, right angled at B. If tan A = `1/sqrt3` , find the value of
- sin A cos C + cos A sin C
- cos A cos C − sin A sin C
If ΔABC, ∠B = 90° and Tan A = `1/sqrt(3)`. Prove that
- Sin A. cos C + cos A. Sin c = 1
- cos A. cos C - sin A. sin C = 0
उत्तर १
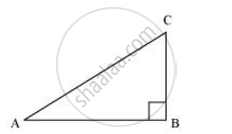
tan A = `1/sqrt3`
`"BC"/"AB"=1/sqrt3`
If BC is k, then AB will be `sqrt3k`, where k is a positive integer.
In ΔABC,
AC2 = AB2 + BC2
= `(sqrt3k)^2 + (k)^2`
= 3k2 + k2
= 4k2
∴ AC = 2k
sin A = `("Side adjacent to ∠A")/"Hypotenuse" = ("BC")/("AC") = k/(2k) = 1/2`
cos A = `("Side adjacent to ∠A")/"Hypotenuse" = ("AB")/("AC") = (sqrt3k)/(2k) = sqrt3/2`
sin C = `("Side adjacent to ∠C")/"Hypotenuse" = ("AB")/("AC") = (sqrt3k)/(2k) = sqrt3/2`
cos C = `("Side adjacent to ∠C")/"Hypotenuse" = ("BC")/("AC") = (k)/(2k) = 1/2`
(i) sin A cos C + cos A sin C
= `(1/2)(1/2)+(sqrt3/2)(sqrt3/2) `
= `1/4 + 3/4`
= `4/4`
= 1
(ii) cos A cos C − sin A sin C
= `(sqrt3/2)(1/2)-(1/2)(sqrt3/2)`
= `sqrt3/4 - sqrt3/4`
= 0
उत्तर २
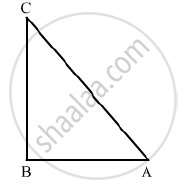
In ΔABC, ∠B = 90°,
As, tan A = `1/sqrt(3)`
⇒ `("BC")/("AB") = 1/sqrt(3)`
Let BC = x and AB = x = `sqrt(3)`
Using Pythagoras the get
AC = `sqrt("AB"^2 + "BC"^2)`
= `sqrt((xsqrt(3))^2 + x^2)`
= `sqrt(3x^2 + x^2)`
= `sqrt(4x^2)`
= 2x
Now,
(i) LHS = sin A. cos C + cos A . sin C
= `("BC")/("AC") . ("BC")/("AC") + ("AB")/("AC") .("AB")/("AC")`
= `(("BC")/("AC"))^2 + (("AB")/("AC"))^2`
= `(x/(2x))^2 + ((xsqrt(3))/(2x))^2`
= `1/4 +3/4`
= 1
= RHS
(ii) LHS = cos A . cos C - sinA . sinC
= `("AB")/("AC") .("BC")/("AC") -("BC")/("AC") .("AB")/("AC")`
= `(xsqrt(3))/(2x) .x/2x - x/2x.(xsqrt(3))/(2x)`
= `sqrt(3)/4 - sqrt(3)/4`
= 0
= RHS
संबंधित प्रश्न
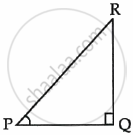
In Given Figure, find tan P – cot R.

State whether the following are true or false. Justify your answer.
sec A = `12/5` for some value of angle A.
If `sin theta = a/b` find sec θ + tan θ in terms of a and b.
If `tan θ = 20/21` show that `(1 - sin theta + cos theta)/(1 + sin theta + cos theta) = 3/7`
Evaluate the Following
`cot^2 30^@ - 2 cos^2 60^circ- 3/4 sec^2 45^@ - 4 sec^2 30^@`
Evaluate the Following
`4/(cot^2 30^@) + 1/(sin^2 60^@) - cos^2 45^@`
Evaluate the Following
4(sin4 30° + cos2 60°) − 3(cos2 45° − sin2 90°) − sin2 60°
Find the value of x in the following :
`2sin 3x = sqrt3`
Find the value of x in the following :
`sqrt3 sin x = cos x`
Find the value of x in the following :
`sqrt3 tan 2x = cos 60^@ + sin45^@ cos 45^@`
If sin (A − B) = sin A cos B − cos A sin B and cos (A − B) = cos A cos B + sin A sin B, find the values of sin 15° and cos 15°.
If cos A + cos² A = 1, then sin² A + sin4 A is equal to ______.
If cos (81 + θ)° = sin`("k"/3 - theta)^circ` where θ is an acute angle, then the value of k is ______.
If sin 2A = `1/2` tan² 45° where A is an acute angle, then the value of A is ______.
If sin θ + sin² θ = 1, then cos² θ + cos4 θ = ______.
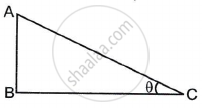
In ΔABC, ∠ABC = 90° and ∠ACB = θ. Then write the ratios of sin θ and tan θ from the figure.
Find the value of sin 45° + cos 45° + tan 45°.
The maximum value of the expression 5cosα + 12sinα – 8 is equal to ______.
In a right triangle PQR, right angled at Q. If tan P = `sqrt(3)`, then evaluate 2 sin P cos P.