Advertisements
Advertisements
प्रश्न
If the medians of a ∆ABC intersect at G, show that ar (AGB) = ar (AGC) = ar (BGC) = `1/3` ar (ABC)
उत्तर
Given: In ΔABC, AD, BE and CF are medians and intersect at G.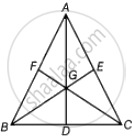
To prove: ar (ΔAGB) = ar (ΔAGC) = ar (ΔBGC) = `1/3` ar (ΔABC)
Proof: We know that, a median of a triangle divides it into two triangles of equal area.
In ΔABC, AD is a median
∴ ar (ΔABD) = ar (ΔACD) ...(i)
In ΔBGC, GD is a median
∴ ar (ΔGBD) = ar (ΔGCD) ...(ii)
On subtracting equation (ii) from equation (i), we get
ar (ΔABD) – ar (ΔGBD) = ar (ΔACD) – ar (ΔGCD)
⇒ ar (ΔAGB) = ar (ΔAGC) ...(iii)
Similarly, ar (ΔAGB) = ar (ΔBGC) ...(iv)
From equations (iii) and (iv),
ar (ΔAGB) = ar (ΔBGC) = ar (ΔAGC) ...(v)
Now, ar (ΔABC) = ar (ΔAGB) + ar (ΔBGC) + ar (ΔAGC)
⇒ ar (ΔABC) = ar (ΔAGB) + ar (ΔAGB) + ar (ΔAGB) ...[From equation (v)]
⇒ ar (ΔABC) = 3 ar (ΔAGB)
⇒ ar (ΔAGB) = `1/3` ar (ΔABC) ...(vi)
From eqattions (v) and (vi),
ar (ΔBGC) = `1/3` ar (ΔABC)
And ar (ΔAGC) = `1/3` ar (ΔABC)
Hence proved.
APPEARS IN
संबंधित प्रश्न
In the given figure, ABC and ABD are two triangles on the same base AB. If line-segment CD is bisected by AB at O, show that ar (ABC) = ar (ABD).
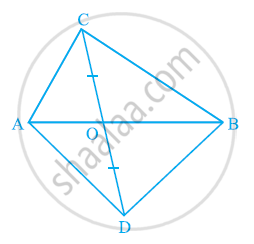
In the given figure, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that:
(i) ar (DOC) = ar (AOB)
(ii) ar (DCB) = ar (ACB)
(iii) DA || CB or ABCD is a parallelogram.
[Hint: From D and B, draw perpendiculars to AC.]

The side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed (see the following figure). Show that
ar (ABCD) = ar (PBQR).
[Hint: Join AC and PQ. Now compare area (ACQ) and area (APQ)]
In a ΔABC, P and Q are respectively the mid-points of AB and BC and R is the mid-point
of AP. Prove that :
(1) ar (Δ PBQ) = ar (Δ ARC)
(2) ar (Δ PRQ) =`1/2`ar (Δ ARC)
(3) ar (Δ RQC) =`3/8` ar (Δ ABC) .
PQRS is a parallelogram whose area is 180 cm2 and A is any point on the diagonal QS. The area of ∆ASR = 90 cm2.
ABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE) = `1/4` ar (ABC).
The area of the parallelogram ABCD is 90 cm2 (see figure). Find ar (ΔBEF)
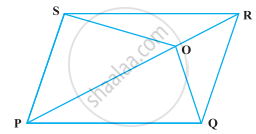
O is any point on the diagonal PR of a parallelogram PQRS (Figure). Prove that ar (PSO) = ar (PQO).
In ∆ABC, if L and M are the points on AB and AC, respectively such that LM || BC. Prove that ar (LOB) = ar (MOC)
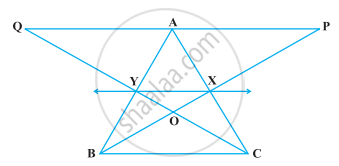
In the following figure, X and Y are the mid-points of AC and AB respectively, QP || BC and CYQ and BXP are straight lines. Prove that ar (ABP) = ar (ACQ).