Advertisements
Advertisements
प्रश्न
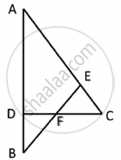
In ΔABC, AB = AC. Side BC is produced to D. Prove that `AD^2−AC^2`= BD.CD
उत्तर
Draw AE⊥BC, meeting BC at D.
Applying Pythagoras theorem in right-angled triangle AED, we get:

Since, ABC is an isosceles triangle and AE is the altitude and we know that the altitude is also the median of the isosceles triangle.
So, BE = CE
And DE+CE=DE+BE=BD
`AD^2=AE^2+DE^2`
`⇒ AE^2=AD^2-DE^2 ` ...............(1)
In ΔACE,
`AC^2=AE^2+EC^2`
⇒ `AE^2=AC^2-EC^2 ` ...............(2)
Using (i) and (ii),
⇒` AD^2-DE^2=AC^2-EC^2`
⇒` AD^2-AC^2=DE^2-EC^2`
`=(DE+CE) (DE-CE)`
`(DE+BE) CD`
`BD.CD`
APPEARS IN
संबंधित प्रश्न
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 8x − 7, DB = 5x − 3, AE = 4x − 3 and EC = (3x − 1), find the value of x.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 5.6cm, AD = 1.4cm, AC= 7.2 cm and AE = 1.8 cm.
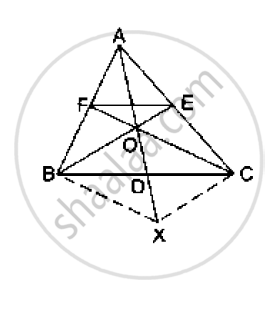
In the given figure, side BC of a ΔABC is bisected at D
and O is any point on AD. BO and CO produced meet
AC and AB at E and F respectively, and AD is
produced to X so that D is the midpoint of OX.
Prove that AO : AX = AF : AB and show that EF║BC.
ΔABC is an isosceles triangle with AB = AC = 13cm. The length of altitude from A on BC is 5cm. Find BC.
ABC is an isosceles triangle, right-angled at B. Similar triangles ACD and ABE are constructed on sides AC and AB. Find the ratio between the areas of ΔABE and ΔACD.
State and converse of Thale’s theorem.
In fig, seg DE || sec BC, identify the correct statement.
In fig., PS = 2, SQ = 6, QR = 5, PT = x and TR = y. Then find the pair of value of x and y such that ST || side QR.
Find the value of x for which DE || AB in figure.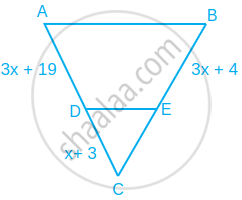
In the given figure ∠CEF = ∠CFE. F is the midpoint of DC. Prove that `(AB)/(BD) = (AE)/(FD)`