Advertisements
Advertisements
प्रश्न
In ∆ABC, AD and BE are altitude. Prove that\[\frac{ar\left( ∆ DEC \right)}{ar\left( ∆ ABC \right)} = \frac{{DC}^2}{{AC}^2}\]
उत्तर
Δ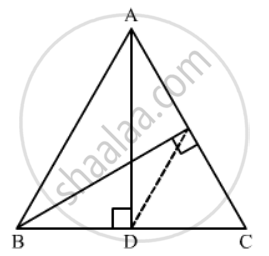
Given: ΔABC in which AD and BE are altitudes on sides BC and AC respectively.
Since ∠ADB = ∠AEB = 90°, there must be a circle passing through point D and E having AB as diameter.
We also know that, angle in a semi-circle is a right angle.
Now, join DE.
So, ABDE is a cyclic quadrilateral with AB being the diameter of the circle.
∠A + ∠BDE = 180° [Opposite angles in a cyclic quadrilateral are supplementary]
⇒ ∠A + (∠BDA + ∠ADE) = 180°
⇒ ∠BDA + ∠ADE = 180° − ∠A ..... (1)
Again,
∠BDA + ∠ADC = 180° [Linear pair]
⇒ ∠BDA + ∠ADE + ∠EDC = 180°
⇒ ∠BDA + ∠ADE = 180° − ∠EDC ..... (2)
Equating (1) and (2), we get
180° − ∠A = 180° − ∠EDC
⇒ ∠A = ∠EDC
Similarly, ∠B = ∠CED
Now, in ΔABC and ΔDEC, we have
∠A = ∠EDC
∠B = ∠CED
∠C = ∠C
∴ ΔABC ∼ ΔDEC
`⇒ \text{(Area of Δ DEC )}/ \text{(Area of Δ ABC )}= ((DC)/(AC))^2`
APPEARS IN
संबंधित प्रश्न
the below given figure, a triangle ABC is drawn to circumscribe a circle of radius 3 cm, such that the segments BD and DC are respectively of lengths 6 cm and 9 cm. If the area of ΔABC is 54 cm2, then find the lengths of sides AB and AC.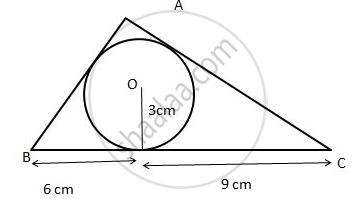
The incircle of an isosceles triangle ABC, in which AB = AC, touches the sides BC, CA and AB at D, E and F respectively. Prove that BD = DC.
In an isosceles ΔABC, the base AB is produced both the ways to P and Q such that AP × BQ = AC2. Prove that ΔAPC ~ ΔBCQ.
In the given figure, DE || BD. Determine AC and AE.

In ∆ABC, given that AB = AC and BD ⊥ AC. Prove that BC2 = 2 AC. CD
If ∆ABC and ∆DEF are two triangles such tha\[\frac{AB}{DE} = \frac{BC}{EF} = \frac{CA}{FD} = \frac{2}{5}\] , then Area (∆ABC) : Area (∆DEF) =
In the given figure, the value of x for which DE || AB is

In the given figure, RS || DB || PQ. If CP = PD = 11 cm and DR = RA = 3 cm. Then the values of x and y are respectively.

If E is a point on side CA of an equilateral triangle ABC such that BE ⊥ CA, then AB2 + BC2 + CA2 =
D and E are respectively the points on the sides AB and AC of a triangle ABC such that AD = 2 cm, BD = 3 cm, BC = 7.5 cm and DE || BC. Then, length of DE (in cm) is ______.
