Advertisements
Advertisements
प्रश्न
In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.
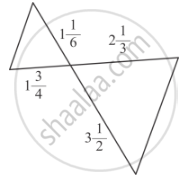
उत्तर
In two triangle, we observe that

`(3(1)/2)/(1(1)/6)=(2(1)/3)/(1(3)/4)`
`(7/2)/(7/6)=(7/3)/(7/4)`
`7/2xx6/7=7/3xx4/7`
`cancel7/2xx6/cancel7=cancel7/3xx4/cancel7`
`cancel(6)^3/cancel2=4/3`
`3=4/3`
In two triangles corresponding sides are not proportional to each other.
NO two triangles are not similar.
APPEARS IN
संबंधित प्रश्न
The incircle of an isosceles triangle ABC, in which AB = AC, touches the sides BC, CA and AB at D, E and F respectively. Prove that BD = DC.
In a ΔABC, AD is the bisector of ∠A.
If AB = 5.6cm, BD = 3.2cm and BC = 6cm, find AC.

In each of the following figures, you find who triangles. Indicate whether the triangles are similar. Give reasons in support of your answer.

ABCD is a trapezium in which AB || DC. P and Q are points on sides AD and BC such that PQ || AB. If PD = 18, BQ = 35 and QC = 15, find AD.
If in two triangles ABC and DEF, \[\frac{AB}{DE} = \frac{BC}{FE} = \frac{CA}{FD}\], then
∆ABC ∼ ∆PQR such that ar(∆ABC) = 4 ar(∆PQR). If BC = 12 cm, then QR =
In an equilateral triangle ABC if AD ⊥ BC, then
In the given figure, if ∠ADE = ∠ABC, then CE =
In a ∆ABC, ∠A = 90°, AB = 5 cm and AC = 12 cm. If AD ⊥ BC, then AD =
∆ABC is such that AB = 3cm, BC = 2cm, CA = 2.5cm. If ∆ABC ~ ∆DEF and EF = 4cm, then perimeter of ∆DEF is ______.
