Advertisements
Advertisements
प्रश्न
In figure, arcs are drawn by taking vertices A, B and C of an equilateral triangle of side 10 cm. to intersect the sides BC, CA and AB at their respective mid-points D, E and F. Find the area of the shaded region (Use π = 3.14).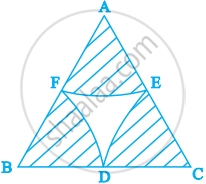
उत्तर
Since, ΔABC is an equilateral triangle.
∴ ∠A = ∠B = ∠C = 60°
And AB = BC = CA = 10 cm
E, F and D are mid-points of the given sides.
∴ AE = EC = CD = DB = BF = FA = 5 cm
Radius of a sector (r) = 5 cm
Now, area of sector CDE
= `θ/360^circ xx π"r"^2`
= `60^circ/360^circ xx 3.14 xx (5)^2 "cm"^2`
= `(3.14 xx 25)/6 "cm"^2`
= `78.5/6 "cm"^2`
= 13.0833 cm2
∴ Area of shaded region
= 3 (Area of sector CDE)
= 3 × 13.0833 cm2
= 39.25 cm2
APPEARS IN
संबंधित प्रश्न
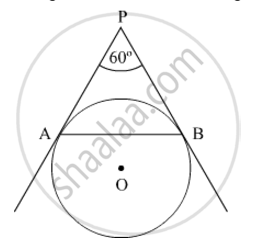
In Fig. 3, AP and BP are tangents to a circle with centre O, such that AP = 5 cm and ∠APB = 60°. Find the length of chord AB.
A horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (see the given figure). Find
- The area of that part of the field in which the horse can graze.
- The increase in the grazing area of the rope were 10 m long instead of 5 m. [Use π = 3.14]

A sector is cut-off from a circle of radius 21 cm the angle of sector is 120°. Find the length of its arc and its area.
A chord 10 cm long is drawn in a circle whose radius is 5√2 cm. Find the area of both
segments
A chord AB of circle, of radius 14cm makes an angle of 60° at the centre. Find the area of minor segment of circle.
In following fig., ABCD is a square. A cirde is drawn with centre A so that it cuts AB and AD at Mand N respectively. Prove that Δ DAM ≅ Δ .BAN.
In following figure , C is a point on the minor arc AB of the circle with centre O . Given ∠ ACB = p° , ∠ AOB = q° , express q in terms of p. Calculate p if OACB is a parallelogram.

The area of the sector of a circle with radius 6 cm and of angle 60° is ____________.
Find the area of the segment of a circle of radius 12 cm whose corresponding sector has a central angle of 60° (Use π = 3.14).
Find the perimeter of a quadrant of a circle of radius 14 cm.
