Advertisements
Advertisements
प्रश्न
In the given figure, PA and PB are the tangent segemtns to a circle with centre O. Show that he points A, O, B and P are concyclic.

उत्तर
Here, OA = OB
And OA ⊥ AP,OA ⊥ BP (Since tangents drawn from an external point are perpendicular to the radius at the point of contact)
∴ ∠ OAP = 90° , ∠ OBP = 90°
∴ ∠OAP +∠ OBP = 90° + 90° = 180°
∴ ∠ AOB + ∠APB =180° (Since, ∠OAP +∠OBP +∠AOB +∠APB = 360° )
Sum of opposite angle of a quadrilateral is 180° .
Hence A, O, B and P are concyclic.
APPEARS IN
संबंधित प्रश्न
Two circles touch each other externally at P. AB is a common tangent to the circles touching them at A and B. The value of ∠ L APB is
(A) 30°
(B) 45°
(C) 60°
(D) 90°
Fill in the blank
The angle between tangent at a point on a circle and the radius through the point is ........
O is the center of a circle of radius 8cm. The tangent at a point A on the circle cuts a line through O at B such that AB = 15 cm. Find OB
If from any point on the common chord of two intersecting circles, tangents be drawn to circles, prove that they are equal.
Two tangent segments PA and PB are drawn to a circle with center O such that ∠APB =120°. Prove that OP = 2AP
In the given figure, BC is a tangent to the circle with centre O. OE bisects AP. Prove that ΔAEO~Δ ABC.

The greatest chord of a circle is called its
The ratio between the circumference and diameter of any circle is _______
In the given figure, O is the centre of the circle. Name all chords of the circle.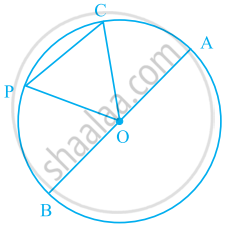
Is every chord of a circle also a diameter?
