Advertisements
Advertisements
प्रश्न
Two circles touch each other externally at P. AB is a common tangent to the circles touching them at A and B. The value of ∠ L APB is
(A) 30°
(B) 45°
(C) 60°
(D) 90°
उत्तर
Correct answer: D
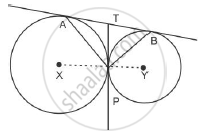
TA = TP ⇒ ∠TAP = ∠TPA
TB = TP ⇒∠TBP = ´TPB
∠TAP + ∠TBP
Correct answer: D

TA = TP ⇒ ∠TAP = ∠TPA
TB = TP ⇒∠TBP = ´TPB
∠TAP + ∠TBP= ∠TPA + ∠TPB= ∠APB
` ∠TAP + ∠TBP+ ∠APB=180^@[because "sum of" ......180^@]`
`/_APB+/_APB=180^@`
`2/_APB=180^@`
`/_APB=90^@`
APPEARS IN
संबंधित प्रश्न
Fill in the blank
The angle between tangent at a point on a circle and the radius through the point is ........
If the tangent at point P to the circle with center O cuts a line through O at Q such that PQ= 24cm and OQ = 25 cm. Find the radius of circle
In the adjoining figure, a circle touches all the four sides of a quadrilateral ABCD whose sides are AB=6cm, BC=9cm and CD=8 cm. Find the length of side AD.

If \[d_1 , d_2 ( d_2 > d_1 )\] be the diameters of two concentric circle s and c be the length of a chord of a circle which is tangent to the other circle , prove that\[{d_2}^2 = c^2 + {d_1}^2\].
Radius of a circle with centre O is 4 cm. If l(OP) = 4.2 cm, say where point P will lie.
Find the length of the chord AC where AB and CD are the two diameters perpendicular to each other of a circle with radius `4sqrt(2)` cm and also find ∠OAC and ∠OCA
In the following figure, if AOB is a diameter of the circle and AC = BC, then ∠CAB is equal to ______.

In the given figure, O is the centre of the circle. Name all radii of the circle.
Is every chord of a circle also a diameter?
The circumcentre of a triangle is the point which is ______.
