Advertisements
Advertisements
प्रश्न
The equation of a circle with origin as centre and passing through the vertices of an equilateral triangle whose median is of length 3a is ______.
पर्याय
x2 + y2 = 9a2
x2 + y2 = 16a2
x2 + y2 = 4a2
x2 + y2 = a2
Advertisements
उत्तर
The equation of a circle with origin as centre and passing through the vertices of an equilateral triangle whose median is of length 3a is x2 + y2 = 4a2.
Explanation: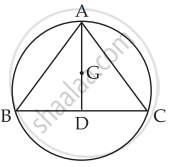
Let ABC be an equilateral triangle in which median AD = 3a.
Centre of the circle is same as the centroid of the triangle
i.e., (0, 0)
AG : GD = 2 : 1
So, AG = `2/3` AD = `2/3 xx 3a = 2a`
∴ The equation of the circle is (x – 0)2 + (y – 0)2 = (2a)2
⇒ x2 + y2 = 4a2
APPEARS IN
संबंधित प्रश्न
Find the equation of the circle with:
Centre (a, b) and radius\[\sqrt{a^2 + b^2}\]
Find the equation of the circle with:
Centre (a, a) and radius \[\sqrt{2}\]a.
Find the centre and radius of each of the following circles:
(x − 1)2 + y2 = 4
Find the centre and radius of each of the following circles:
x2 + y2 − 4x + 6y = 5
Find the equation of the circle whose centre is (1, 2) and which passes through the point (4, 6).
Find the equation of the circle whose centre lies on the positive direction of y - axis at a distance 6 from the origin and whose radius is 4.
Find the equation of a circle
which touches both the axes and passes through the point (2, 1).
Find the equation of the circle which touches the axes and whose centre lies on x − 2y = 3.
Find the equations of the circles touching y-axis at (0, 3) and making an intercept of 8 units on the X-axis.
If the lines 2x − 3y = 5 and 3x − 4y = 7 are the diameters of a circle of area 154 square units, then obtain the equation of the circle.
If the lines 3x − 4y + 4 = 0 and 6x − 8y − 7 = 0 are tangents to a circle, then find the radius of the circle.
Show that the point (x, y) given by \[x = \frac{2at}{1 + t^2}\] and \[y = a\left( \frac{1 - t^2}{1 + t^2} \right)\] lies on a circle for all real values of t such that \[- 1 \leq t \leq 1\] where a is any given real number.
One diameter of the circle circumscribing the rectangle ABCD is 4y = x + 7. If the coordinates of A and B are (−3, 4) and (5, 4) respectively, find the equation of the circle.
Find the coordinates of the centre and radius of the following circle:
1/2 (x2 + y2) + x cos θ + y sin θ − 4 = 0
Find the equation of the circle passing through the points:
(5, 7), (8, 1) and (1, 3)
Find the equation of the circle passing through the points:
(5, −8), (−2, 9) and (2, 1)
Find the equation of the circle which passes through (3, −2), (−2, 0) and has its centre on the line 2x − y = 3.
Show that the points (5, 5), (6, 4), (−2, 4) and (7, 1) all lie on a circle, and find its equation, centre and radius.
Find the equation of the circle which circumscribes the triangle formed by the lines x + y + 3 = 0, x − y + 1 = 0 and x = 3
Find the equation of the circle which circumscribes the triangle formed by the lines 2x + y − 3 = 0, x + y − 1 = 0 and 3x + 2y − 5 = 0
Find the equation of the circle which passes through the origin and cuts off chords of lengths 4 and 6 on the positive side of the x-axis and y-axis respectively.
Find the equation of the circle concentric with the circle x2 + y2 − 6x + 12y + 15 = 0 and double of its area.
Find the equation to the circle which passes through the points (1, 1) (2, 2) and whose radius is 1. Show that there are two such circles.
Find the equation of the circle concentric with x2 + y2 − 4x − 6y − 3 = 0 and which touches the y-axis.
Find the equation of the circle, the end points of whose diameter are (2, −3) and (−2, 4). Find its centre and radius.
The sides of a square are x = 6, x = 9, y = 3 and y = 6. Find the equation of a circle drawn on the diagonal of the square as its diameter.
Find the equation of the circle circumscribing the rectangle whose sides are x − 3y = 4, 3x + y = 22, x − 3y = 14 and 3x + y = 62.
Find the equations of the circles which pass through the origin and cut off equal chords of \[\sqrt{2}\] units from the lines y = x and y = − x.
If the abscissae and ordinates of two points P and Q are roots of the equations x2 + 2ax − b2 = 0 and x2 + 2px − q2 = 0 respectively, then write the equation of the circle with PQ as diameter.
If the equation of a circle is λx2 + (2λ − 3) y2 − 4x + 6y − 1 = 0, then the coordinates of centre are
If 2x2 + λxy + 2y2 + (λ − 4) x + 6y − 5 = 0 is the equation of a circle, then its radius is
The equation of the circle passing through the point (1, 1) and having two diameters along the pair of lines x2 − y2 −2x + 4y − 3 = 0, is
If the centroid of an equilateral triangle is (1, 1) and its one vertex is (−1, 2), then the equation of its circumcircle is
If the point (λ, λ + 1) lies inside the region bounded by the curve \[x = \sqrt{25 - y^2}\] and y-axis, then λ belongs to the interval
The equation of the circle which touches the axes of coordinates and the line \[\frac{x}{3} + \frac{y}{4} = 1\] and whose centres lie in the first quadrant is x2 + y2 − 2cx − 2cy + c2 = 0, where c is equal to
If the circles x2 + y2 = a and x2 + y2 − 6x − 8y + 9 = 0, touch externally, then a =
Equation of the circle with centre on the y-axis and passing through the origin and the point (2, 3) is ______.
