Advertisements
Advertisements
प्रश्न
The length of a shadow of a tower standing on a level plane is found to be 2y meters longer when the seen's altitude is 30° than when it was 45° prove that the height of the tower is y ( √3 + 1 ) meter.
उत्तर
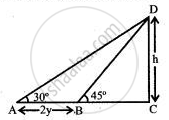
In the right-angled triangle BCD.
tan 45° = `h/(BC)`
h = BC ....(1)
In right-angled Δ ACD,
tan 30° = `h/(2y + BC)`
⇒ `1/sqrt3 = h/(2y + h)`
⇒ `h(sqrt3 - 1) = 2y`
⇒ h = y ( √3 + 1 ) m
Hence proved.
APPEARS IN
संबंधित प्रश्न
Prove the following identities, where the angles involved are acute angles for which the expressions are defined:
`((1+tan^2A)/(1+cot^2A))=((1-tanA)/(1-cotA))^2=tan^2A`
Without using trigonometric tables, evaluate :
`sin 16^circ/cos 74^circ`
Without using trigonometric tables, evaluate :
`tan 27^circ/cot 63^circ`
Without using trigonometric tables, evaluate :
`("cosec" 42^circ)/sec 48^circ`
Without using trigonometric tables, evaluate :
`cot 38^circ/tan 52^circ`
Without using trigonometric tables, prove that:
cosec 80° − sec 10° = 0
Without using trigonometric tables, prove that:
cos275° + cos215° = 1
Without using trigonometric tables, prove that:
sin53° cos37° + cos53° sin37° = 1
If sec 4 A = cosec (A − 15°), where 4 A is an acute angle, find the value of A.
