Advertisements
Advertisements
Question
The length of a shadow of a tower standing on a level plane is found to be 2y meters longer when the seen's altitude is 30° than when it was 45° prove that the height of the tower is y ( √3 + 1 ) meter.
Solution
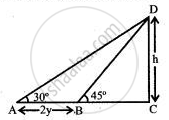
In the right-angled triangle BCD.
tan 45° = `h/(BC)`
h = BC ....(1)
In right-angled Δ ACD,
tan 30° = `h/(2y + BC)`
⇒ `1/sqrt3 = h/(2y + h)`
⇒ `h(sqrt3 - 1) = 2y`
⇒ h = y ( √3 + 1 ) m
Hence proved.
APPEARS IN
RELATED QUESTIONS
In the below given figure, a tower AB is 20 m high and BC, its shadow on the ground, is 20√3 m long. Find the sun’s altitude.
Evaluate without using trigonometric tables,
`sin^2 28^@ + sin^2 62^@ + tan^2 38^@ - cot^2 52^@ + 1/4 sec^2 30^@`
Without using trigonometrical tables, evaluate:
`cosec^2 57^circ - tan^2 33^circ + cos 44^circ cosec 46^circ - sqrt(2) cos 45^circ - tan^2 60^circ`
Without using trigonometric tables, prove that:
(sin72° + cos18°)(sin72° − cos18°) = 0
Prove that:
`(sin 70^circ)/(cos 20^circ) + ("cosec" 20^circ)/(sec 70^circ) - 2 cos 70^circ "cosec" 20^circ = 0`
Prove that:
\[\frac{\sin\theta \cos(90^\circ - \theta)\cos\theta}{\sin(90^\circ- \theta)} + \frac{\cos\theta \sin(90^\circ - \theta)\sin\theta}{\cos(90^\circ - \theta)}\]
From the trigonometric table, write the values of tan 45°48'.
Solve the following equation: `(cos^2θ - 3 cosθ + 2)/sin^2θ` = 1.
Using trigonometric table evaluate the following:
tan 25°45' + cot 45°25'.
Given that sin θ = `a/b` then cos θ is equal to ______.
