Advertisements
Advertisements
प्रश्न
Two adjacent sides of a parallelogram are 28 cm and 26 cm. If one diagonal of it is 30 cm long; find the area of the parallelogram. Also, find the distance between its shorter sides.
उत्तर
At first, we have to calculate the area of the triangle having sides, then its perimeter 28 cm, 26 cm, and 30 cm.
Let a = 28, b = 26, c = 30
S = `[ 28 + 26 + 30 ]/2`
= `84/2`
= 42 cm
By Heron's Formula,
Area of a triangle = `sqrt[s( s - a )( s - b )( s - c )]`
= `sqrt[42( 42 - 28 )( 42 - 26 )( 42 - 30 )]`
= `sqrt( 42 xx 14 xx 16 xx 12)`
= `sqrt( 112896 )`
= 336 cm2
Area of a Parallelogram = 2 × Area of a triangle
= 2 × 336
= 672 cm2
We know that,
Area of a parallelogram = Height × Base
⇒ 672 = Height × 26
⇒ Height = 25.84 cm
∴ The distance between its shorter sides is 25.84 cm.
APPEARS IN
संबंधित प्रश्न
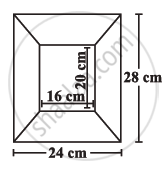
Diagram of the adjacent picture frame has outer dimensions = 24 cm × 28 cm and inner dimensions 16 cm × 20 cm. Find the area of each section of the frame, if the width of each section is same.
Find the area of a quadrilateral one of whose diagonals is 30 cm long and the perpendiculars from the other two vertices are 19 cm and 11 cm respectively.
The diagonals of a quadrilateral are 16 cm and 13 cm. If they intersect each other at right angles; find the area of the quadrilateral.
Trapezium given below; find its area.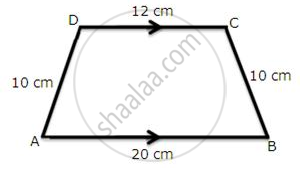
Trapezium given below; find its area.

A footpath of uniform width runs all around the outside of a rectangular field 30 m long and 24 m wide. If the path occupies an area of 360 m2, find its width.
The perimeter of a rhombus is 52 cm. If one diagonal is 24 cm; find:
(i) The length of its other diagonal,
(ii) Its area.
Vertices of given triangles are taken in order and their areas are provided aside. Find the value of ‘p’.
| Vertices | Area (sq.units) |
| (p, p), (5, 6), (5, –2) | 32 |
When proving that a quadrilateral is a parallelogram by using slopes you must find
If the diagonal d of a quadrilateral is doubled and the heights h1 and h2 falling on d are halved, then the area of quadrilateral is ______.
