Advertisements
Advertisements
प्रश्न
Two poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.
उत्तर
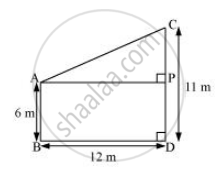
Let CD and AB be the poles of height 11 m and 6 m.
Therefore, CP = 11 − 6 = 5 m
From the figure, it can be observed that AP = 12m
Applying Pythagoras theorem for ΔAPC, we obtain
AP2 + PC2 = AC2
(12 m)2 + (5m)2 = (AC)2
AC2 = (144 + 25)m2 = 169 m2
AC = 13m
Therefore, the distance between their tops is 13 m.
संबंधित प्रश्न
In the given figure, ABC is a triangle in which ∠ABC < 90° and AD ⊥ BC. Prove that AC2 = AB2 + BC2 − 2BC.BD.

Which of the following can be the sides of a right triangle?
2.5 cm, 6.5 cm, 6 cm
In the case of right-angled triangles, identify the right angles.
Identify, with reason, if the following is a Pythagorean triplet.
(11, 60, 61)
The sides of a certain triangle is given below. Find, which of them is right-triangle
6 m, 9 m, and 13 m
In triangle PQR, angle Q = 90°, find: PR, if PQ = 8 cm and QR = 6 cm
In the given figure, angle ACB = 90° = angle ACD. If AB = 10 m, BC = 6 cm and AD = 17 cm, find :
(i) AC
(ii) CD

Use the information given in the figure to find the length AD.

The sides of the triangle are given below. Find out which one is the right-angled triangle?
40, 20, 30
In ∆PQR, PD ⊥ QR such that D lies on QR. If PQ = a, PR = b, QD = c and DR = d, prove that (a + b)(a – b) = (c + d)(c – d).
Height of a pole is 8 m. Find the length of rope tied with its top from a point on the ground at a distance of 6 m from its bottom.
