Advertisements
Advertisements
Question
A(5, x), B(−4, 3) and C(y, –2) are the vertices of the triangle ABC whose centroid is the origin. Calculate the values of x and y.
Solution
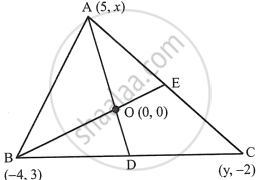
Co-ordinates of centroid of ΔABC are (0, 0)
∴ `0 = (x_1 + x_2 + x_3)/3`
= `(5 - 4 + y)/3`
`=> 0 = (y + 1)/3`
`=>` y + 1 = 0
∴ y = –1
Again `0 = (y_1 + y_2 + y_3)/3`
= `(x + 3 - 2)/3`
`=> 0 = (x + 1)/3`
`=>` x + 1 = 0
∴ x = –1
∴ x = –1, y = –1
APPEARS IN
RELATED QUESTIONS
One end of the diameter of a circle is (–2, 5). Find the co-ordinates of the other end of it, if the centre of the circle is (2, –1).
In the following example find the co-ordinate of point A which divides segment PQ in the ratio a : b.
P(2, 6), Q(–4, 1), a : b = 1 : 2
The midpoint of the line segment joining the points P (2 , m) and Q (n , 4) is R (3 , 5) . Find the values of m and n.
AB is a diameter of a circle with centre 0. If the ooordinates of A and 0 are ( 1, 4) and (3, 6 ). Find the ooordinates of B and the length of the diameter.
A(3, 1), B(y, 4) and C(1, x) are vertices of a triangle ABC. P, Q and R are mid - points of sides BC, CA and AB respectively. Show that the centroid of ΔPQR is the same as the centroid ΔABC.
Find the mid-point of the line segment joining the points
(−2, 3) and (−6, −5)
If the mid-point (x, y) of the line joining (3, 4) and (p, 7) lies on 2x + 2y + 1 = 0, then what will be the value of p?
If (1, −2), (3, 6), (x, 10) and (3, 2) are the vertices of the parallelogram taken in order, then the value of x is
Find coordinates of midpoint of the segment joining points (0, 2) and (12, 14)
Point P is the centre of the circle and AB is a diameter. Find the coordinates of points B if coordinates of point A and P are (2, – 3) and (– 2, 0) respectively.
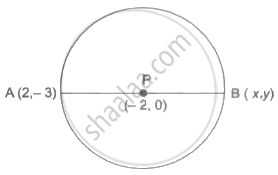
Given: A`square` and P`square`. Let B (x, y)
The centre of the circle is the midpoint of the diameter.
∴ Mid point formula,
`square = (square + x)/square`
⇒ `square = square` + x
⇒ x = `square - square`
⇒ x = – 6
and `square = (square + y)/2`
⇒ `square` + y = 0
⇒ y = 3
Hence coordinates of B is (– 6, 3).
