Advertisements
Advertisements
Question
A 7m wide road surrounds a circular garden whose area is 5544m2. Find the area of the road and the cost of tarring it at the rate of Rs.150 per m2.
Solution
we know,
The area of the ring between two concentric circles equals the area of the larger circle minus the area of smaller circle.
Let the radius of the outer and inner ring be R and r respectively.
Here the circular garden is the inner circle and the 7m wide road os the ring area of the ring between two concentric circles = π(R2 - r2)
The Area of a Circle with radius r = πr2
Here,
πr2 = 5544m2
⇒ r = 42
⇒ R = 42 + 7
= 49
inner Circle has radius r
= 42 and outer Circle has radius R
= 49
π(492 - 422)
= `(22)/(7) xx (2401 - 1764)`
= `(22)/(7) xx 637`
= 2002m2
The cost of paying the road at the rate of Rs.150per m2
= 2002 x 150
= Rs.3,00,300.
APPEARS IN
RELATED QUESTIONS
The cost of painting the four walls of a room 12 m long at `₹ 30 per m^2 is ₹ 7560 per m^2` and the cost of covering the floor with the mat at ₹` 25 per m^2 is ₹ 2700 `. find the dimensions of the room.
Find the area of a parallelogram with base equal to 25 cm and the corresponding height measuring 16.8 cm.
The area of sector is one-twelfth that of the complete circle. Find the angle of the sector .
A circle is inscribed in an equilateral triangle ABC is side 12 cm, touching its sides (the following figure). Find the radius of the inscribed circle and the area of the shaded part.

How many revolutions a circular wheel of radius r metres makes in covering a distance of s metres?
Find the area of the shaded region in the given figure, where a circular arc of radius 6 cm has been drawn with vertex of an equilateral triangle of side 12 cm as centre and a sector of circle of radius 6 cm with centre B is made.
Two circles touch each other externally. The sum of their areas is 58π cm2 and the distance between their centers is 10 cm. Find the radii of the two circles.
The diameters of three wheels are in the ratio 2 : 4 : 8. If the sum of the circumferences of these circles be 132 cm, find the difference between the areas of the largest and the smallest of these wheels.
The sum of diameters of two circles is 112cm and the sum of their areas is 5236cm2. Find the radii of the two circles.
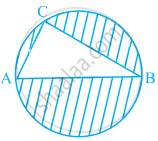
In figure, AB is a diameter of the circle, AC = 6 cm and BC = 8 cm. Find the area of the shaded region (Use π = 3.14).