Advertisements
Advertisements
Question
A child makes a poster on a chart paper drawing a square ABCD of side 14 cm. She draws four circles with centre A, B, C and D in which she suggests different ways to save energy. The circles are drawn in such a way that each circle touches externally two of the three remaining circles (in the following figure). In the shaded region she write a message 'Save Energy'. Find the perimeter and area of the shaded region.
(Use π = 22/7)

Solution
Perimeter of the shaded portion = 4 ⨯ Length of the arc having central angle 90∘
\[= 4 \times \frac{90°}{360°} \times 2 \times \frac{22}{7} \times \frac{14}{2}\]
\[ = 4 \times \frac{1}{4} \times 2 \times \frac{22}{7} \times 7\]
\[ = 44 cm\]
Area of shaded portion = Area of square ABCD − 4 ⨯ Area of the arc having central angle 90∘
\[= \left( 14 \right)^2 - 4 \times \frac{90°}{360°} \times \frac{22}{7} \times \left( \frac{14}{2} \right)^2 \]
\[ = 196 - 4 \times \frac{1}{4} \times \frac{22}{7} \times \left( 7 \right)^2 \]
\[ = 196 - 154\]
\[ = 42 {cm}^2\]
APPEARS IN
RELATED QUESTIONS
The floor of a rectangular hall is 24 m long and 18 m wide. How many carpets, each of length 2.5 m and breadth 80 cm, will be required to cover the floor of the hall?
A footpath of uniform width runs all around the inside of a rectangular field 54m long and 35 m wide. If the area of the path is `420m^2` , find the width of the path.
In the following figure, ABC is a right angled triangle in which ∠A = 90°, AB = 21 cm and AC = 28 cm. Semi-circles are described on AB, BC and AC as diameters. Find the area of the shaded region.
In the following figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π =3.142 and`sqrt3` = 1.732).

Find the area of the largest triangle that can be inscribed in a semi-circle of radius runits.
If the sum of the areas of two circles with radii r1 and r2 is equal to the area of a circle of radius r, then \[r_1^2 + r_2^2\]
Find the area of the shaded region in the given figure, where a circular arc of radius 6 cm has been drawn with vertex of an equilateral triangle of side 12 cm as centre and a sector of circle of radius 6 cm with centre B is made.
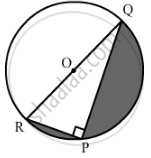
In the given figure, PQ = 24, PR = 7 cm and O is the centre of the circle. Find the area of the shaded region.
The sum of the radii of two circles is 10.5 cm and the difference of their circumferences is 13.2 cm. Find the radii of the two circles.
Thenmozhi wants to level her circular flower garden whose diameter is 49 m at the rate of ₹ 150 per m2 Find the cost of levelling
