Advertisements
Advertisements
Question
A person is standing on a truck moving with a constant velocity of 14.7 m/s on a horizontal road. The man throws a ball in such a way that it returns to the truck after the truck has moved 58.8 m. Find the speed and the angle of projection as seen from the road.
Solution
Given:
Velocity of the truck = 14.7 m/s
Distance covered by the truck when the ball returns again to the truck = 58.8 m
From the road, the motion of ball seems to be a projectile motion.
Total time of flight (T) = 4 seconds
Horizontal range covered by the ball in this time, R = 58.8 m
We know:
R = u cos αt
Here, α is the angle of projection.
Now,
u cos α = 14.7 ...(i)
Now, take the vertical component of velocity.
Using the equation of motion, we get:
Thus, we get:
\[ = 19 . 6 \text{ m } \]
\[ \Rightarrow 19 . 6 = u\sin\alpha\left( 2 \right) - \frac{1}{2} \times 9 . 8 \times 2^2 \]
\[ \Rightarrow 2 u \text{ sin } \alpha = 19 . 6 \times 2\]
\[ \Rightarrow u\sin\alpha = 19 . 6 . . . \left(\text{ ii } \right)\]
Dividing (ii) by (i), we get:
\[\frac{u\sin\alpha}{u\cos\alpha} = \frac{19 . 6}{14 . 7}\]
\[ \Rightarrow \tan\alpha = 1 . 333\]
\[\alpha = \tan^{- 1} (1 . 333)\]
\[ \Rightarrow \alpha = 53^\circ\]
From (i), we get:
u cos α = 14.7
\[\Rightarrow u = \frac{14 . 7}{\cos53^\circ} = 24 . 42 \text{ m } /s \approx 25 \text{ m } /s\]
Therefore, when seen from the road, the speed of the ball is 25 m/s and the angle of projection is 53° with horizontal.
APPEARS IN
RELATED QUESTIONS
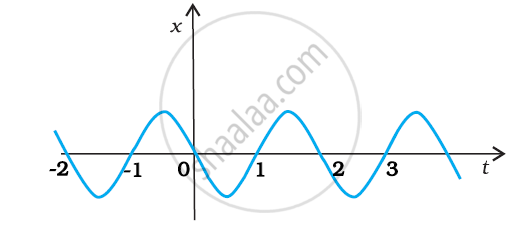
The following figure gives the x-t plot of a particle executing one-dimensional simple harmonic motion. Give the signs of position, velocity and acceleration variables of the particle at t = 0.3 s, 1.2 s, – 1.2 s.
A boy standing on a stationary lift (open from above) throws a ball upwards with the maximum initial speed he can, equal to 49 m/s. How much time does the ball take to return to his hands? If the lift starts moving up with a uniform speed of 5 m/s and the boy again throws the ball up with the maximum speed he can, how long does the ball take to return to his hands?
Two bullets are fired simultaneously, horizontally and with different speeds from the same place. Which bullet will hit he ground first?
A train starts from rest and moves with a constant acceleration of 2.0 m/s2 for half a minute. The brakes are then applied and the train comes to rest in one minute. Find the maximum speed attained by the train .
A bullet going with speed 350 m/s enters a concrete wall and penetrates a distance of 5.0 cm before coming to rest. Find the deceleration.
A particle starting from rest moves with constant acceleration. If it takes 5.0 s to reach the speed 18.0 km/h find the average velocity during this period .
A driver takes 0.20 s to apply the brakes after he sees a need for it. This is called the reaction time of the driver. If he is driving a car at a speed of 54 km/h and the brakes cause a deceleration of 6.0 m/s2, find the distance travelled by the car after he sees the need to put the brakes on.
A ball is projected vertically upward with a speed of 50 m/s. Find the maximum height.
A stone is thrown vertically upward with a speed of 28 m/s.Find its velocity one second before it reaches the maximum height.
A healthy youngman standing at a distance of 7 m from a 11.8 m high building sees a kid slipping from the top floor. With what speed (assumed uniform) should he run to catch the kid at the arms height (1.8 m)?
An elevator is descending with uniform acceleration. To measure the acceleration, a person in the elevator drops a coin at the moment the elevator starts. The coin is 6 ft above the floor of the elevator at the time it is dropped. The person observes that the coin strikes the floor in 1 second. Calculate from these data the acceleration of the elevator.
A ball is thrown at a speed of 40 m/s at an angle of 60° with the horizontal. Find the maximum height reached .
A ball is thrown at a speed of 40 m/s at an angle of 60° with the horizontal. Find the range of the ball. Take g = 10 m/s2.
A person is standing on a truck moving with a constant velocity of 14.7 m/s on a horizontal road. The man throws a ball in such a way that it returns to the truck after the truck has moved 58.8 m. Find the speed and the angle of projection as seen from the truck .
A river 400 m wide is flowing at a rate of 2.0 m/s. A boat is sailing at a velocity of 10 m/s with respect to the water, in a direction perpendicular to the river. Find the time taken by the boat to reach the opposite bank.
A swimmer wishes to cross a 500 m wide river flowing at 5 km/h. His speed with respect to water is 3 km/h. If he heads in a direction making an angle θ with the flow, find the time he takes to cross the river.
Two friends A and B are standing a distance x apart in an open field and wind is blowing from A to B. A beat a drum and B hears the sound t1 time after he sees the event. A and B interchange their positions and the experiment is repeated. This time B hears the drum timer after he sees the event. Calculate the velocity of sound in still air v and the velocity of wind u. Neglect the time light takes in travelling between the friends.
Suppose A and B in the previous problem change their positions in such a way that the line joining them becomes perpendicular to the direction of wind while maintaining the separation x. What will be the time B finds between seeing and hearing the drum beating by A?
