Advertisements
Advertisements
Question
A transverse harmonic wave on a string is described by y(x, t) = 3.0 sin (36t + 0.018x + π/4) where x and y are in cm and t is in s. The positive direction of x is from left to right.
- The wave is travelling from right to left.
- The speed of the wave is 20 m/s.
- Frequency of the wave is 5.7 Hz.
- The least distance between two successive crests in the wave is 2.5 cm.
Solution
a, b and c
Explanation:
The general equation of a plane progressive wave with initial phase is
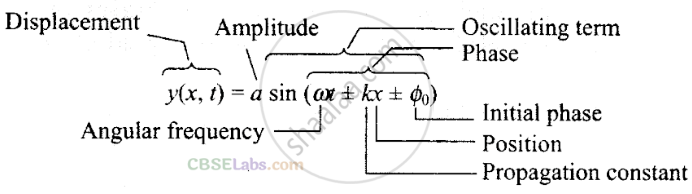
Various forms of the progressive wave function:
- `y = a sin (ωt - kx)`
- `y = a sin (ωt - (2π)/λ x)`
- `y = a sin 2π [t/T - x/λ]`
- `y = a sin (2π)/T (t - x T/λ)`
- `y = a sin (2π)/λ (vt - x)`
- `y = a sin ω(t - x/v)`
Given equation is `y(x, t) = 3.0 sin(36t + 0.018x + π/4)`
Option (a): Since there is +ve sign between wr and kx, the wave travels from right to left (the positive direction of x is from left to right). Hence it is correct.
Option (b): Speed of the wave, `v = ω/k = 36^-1/(0.018 cm)` = 2000 cm/s = 20 m/s. Hence it is correct.
Option (c): Frequency of the wave, `v = ω/(2π) = (36 s^-1)/(2π)` = 5.7 Hz. Hence it is correct.
Option (d): Least distance between two successive crests, `λ = (2π)/k = (2π)/(0.018 cm^-1)` = 349 cm. Hence it is wrong.
APPEARS IN
RELATED QUESTIONS
You have learnt that a travelling wave in one dimension is represented by a function y= f (x, t)where x and t must appear in the combination x – v t or x + v t, i.e. y = f (x ± v t). Is the converse true? Examine if the following functions for y can possibly represent a travelling wave:
(a) `(x – vt )^2`
(b) `log [(x + vt)/x_0]`
(c) `1/(x + vt)`
(i) For the wave on a string described in Exercise 15.11, do all the points on the string oscillate with the same (a) frequency, (b) phase, (c) amplitude? Explain your answers. (ii) What is the amplitude of a point 0.375 m away from one end?
Two wave pulses travel in opposite directions on a string and approach each other. The shape of one pulse is inverted with respect to the other.
Two sine waves travel in the same direction in a medium. The amplitude of each wave is A and the phase difference between the two waves is 120°. The resultant amplitude will be
A wave propagates on a string in the positive x-direction at a velocity \[\nu\] \[t = t_0\] is given by \[g\left( x, t_0 \right) = A \sin \left( x/a \right)\]. Write the wave equation for a general time t.
The equation of a wave travelling on a string is \[y = \left( 0 \cdot 10 \text{ mm } \right) \sin\left[ \left( 31 \cdot 4 m^{- 1} \right)x + \left( 314 s^{- 1} \right)t \right]\]
(a) In which direction does the wave travel? (b) Find the wave speed, the wavelength and the frequency of the wave. (c) What is the maximum displacement and the maximum speed of a portion of the string?
A wave travelling on a string at a speed of 10 m s−1 causes each particle of the string to oscillate with a time period of 20 ms. (a) What is the wavelength of the wave? (b) If the displacement of a particle of 1⋅5 mm at a certain instant, what will be the displacement of a particle 10 cm away from it at the same instant?
An organ pipe of length 0.4 m is open at both ends. The speed of sound in the air is 340 m/s. The fundamental frequency is ______
What is the interference of sound waves?
At what temperatures (in °C) will the speed of sound in air be 3 times its value at O°C?
