Advertisements
Advertisements
Question
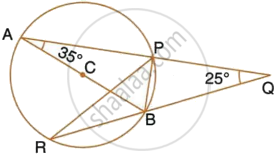
AB is a diameter of the circle APBR as shown in the figure. APQ and RBQ are straight lines. Find : ∠PBR
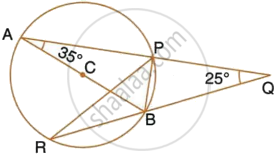
Solution

∠BPA = 90°
(Angle in a semicircle is a right angle)
∴ ∠BPQ = 90°
∴ ∠PBR = ∠BQP + ∠BPQ
= 25° + 90°
= 115°
(Exterior angle of a ∆ is equal to the sum of pair of interior opposite angles)
APPEARS IN
RELATED QUESTIONS
In the given figure, ∠BAD = 65°, ∠ABD = 70°, ∠BDC = 45°
- Prove that AC is a diameter of the circle.
- Find ∠ACB.

If two sides of a cyclic quadrilateral are parallel; prove that:
- its other two sides are equal.
- its diagonals are equal.
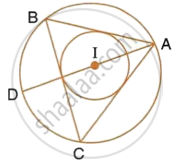
If I is the incentre of triangle ABC and AI when produced meets the circumcircle of triangle ABC in point D. If ∠BAC = 66° and ∠ABC = 80°.
Calculate:
- ∠DBC,
- ∠IBC,
- ∠BIC.
In the given figure, ∠ACE = 43° and ∠CAF = 62°; find the values of a, b and c.

In the figure, given below, CP bisects angle ACB. Show that DP bisects angle ADB.

In the figure given alongside, AB and CD are straight lines through the centre O of a circle. If ∠AOC = 80° and ∠CDE = 40°, find the number of degrees in ∠ABC.

AB is a diameter of the circle APBR, as shown in the figure. APQ and RBQ are straight lines. Find : ∠BPR
If I is the incentre of triangle ABC and AI when produced meets the cicrumcircle of triangle ABC in points D. f ∠BAC = 66° and ∠ABC = 80°. Calculate : ∠BIC.
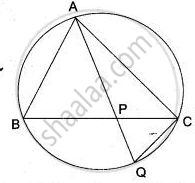
In the given Figure. P is any point on the chord BC of a circle such that AB = AP. Prove that CP = CQ.
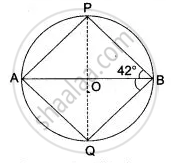
In the following figure, O is the centre of the circle, ∠ PBA = 42°.
Calculate:
(i) ∠ APB
(ii) ∠PQB
(iii) ∠ AQB