Advertisements
Advertisements
Question
ABCD is a kite having AB = AD and BC = CD. Prove that the figure formed by joining the
mid-points of the sides, in order, is a rectangle.
Solution

Given,
A kite ABCD having AB = AD and BC = CD. P,Q,R, S are the midpoint of sides
AB,BC,CD andDArespectively PQ,QR,RS and spare joined
To prove:
PQRS is a rectangle
Proof:
In ΔABC, P and Q are the midpoints of AB and BC respectively.
∴PQ || AC and PQ = `1/2` AC ....(i )
In Δ ADC, R and S are the midpoint of CD and AD respectively.
∴ RS || AC and RS = `1/2` AC .....(ii )
From (i) and (ii), we have
PQ || RS and PQ = RS
Thus, in quadrilateral PQRS, a pair of opposite sides are equal and parallel. So PQRS is a
parallelogram. Now, we shall prove that one angle of parallelogram PQRS it is a right angle
Since AB = AD
⇒ `1/2` AB = AD `(1/2)`
⇒ AP = AS ...(iii) [∵ P and S are the midpoints of B and AD respectively]
⇒ `∠`1 = `∠`2 ....(iv)
Now, in ΔPBQ and ΔSDR, we have
PB = SD [ ∴AD = AB ⇒ `1/2` AD = `1/2` AB ]
BQ = DR ∴ PB = SD
And PQ = SR [ ∴ PQRS is a parallelogram]
So by SSS criterion of congruence, we have
Δ PBQ ≅ Δ SOR
⇒ `∠`3 = `∠`4 [CPCT ]
Now, `∠`3 + `∠`SPQ + `∠`2 = 180°
And `∠`1+ `∠`PSR + `∠`4 =180°
∴ `∠`3 + `∠`SPQ + `∠`2 = `∠`1+ `∠`PSR + `∠`4
⇒ `∠`SPQ = `∠`PSR (`∠`1 = `∠`2 and `∠`3 = `∠`4)
Now, transversal PS cuts parallel lines SR and PQ at S and P respectively.
∴ `∠`SPQ + `∠`PSR = 180°
⇒ 2`∠`SPQ = 180° = `∠`SPQ = 90° [ ∵ `∠`PSR = `∠`SPQ]
Thus, PQRS is a parallelogram such that `∠`SPQ = 90°
Hence, PQRS is a parallelogram.
APPEARS IN
RELATED QUESTIONS
ABC is a triangle and through A, B, C lines are drawn parallel to BC, CA and AB respectively
intersecting at P, Q and R. Prove that the perimeter of ΔPQR is double the perimeter of
ΔABC
In the given figure, `square`PQRS and `square`MNRL are rectangles. If point M is the midpoint of side PR then prove that,
- SL = LR
- LN = `1/2`SQ

The following figure shows a trapezium ABCD in which AB // DC. P is the mid-point of AD and PR // AB. Prove that:
PR = `[1]/[2]` ( AB + CD)

In triangle ABC, angle B is obtuse. D and E are mid-points of sides AB and BC respectively and F is a point on side AC such that EF is parallel to AB. Show that BEFD is a parallelogram.
In a parallelogram ABCD, M is the mid-point AC. X and Y are the points on AB and DC respectively such that AX = CY. Prove that:
(i) Triangle AXM is congruent to triangle CYM, and
(ii) XMY is a straight line.
Show that the quadrilateral formed by joining the mid-points of the adjacent sides of a square is also a square.
In ΔABC, X is the mid-point of AB, and Y is the mid-point of AC. BY and CX are produced and meet the straight line through A parallel to BC at P and Q respectively. Prove AP = AQ.
In ΔABC, the medians BE and CD are produced to the points P and Q respectively such that BE = EP and CD = DQ. Prove that: A is the mid-point of PQ.
In AABC, D and E are two points on the side AB such that AD = DE = EB. Through D and E, lines are drawn parallel to BC which meet the side AC at points F and G respectively. Through F and G, lines are drawn parallel to AB which meet the side BC at points M and N respectively. Prove that BM = MN = NC.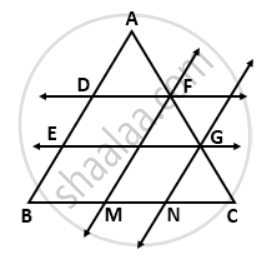
In the given figure, PS = 3RS. M is the midpoint of QR. If TR || MN || QP, then prove that: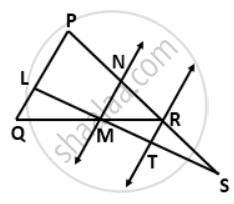
RT = `(1)/(3)"PQ"`
