Advertisements
Advertisements
Question
Derive an expression for the net torque on a rectangular current carrying loop placed in a uniform magnetic field with its rotational axis perpendicular to the field.
Solution
- Consider rectangular loop abcd placed in a uniform magnetic field `vec"B"` such that the sides ab and cd are perpendicular to the magnetic field `vec"B"` but the sides bc and da are not, as shown in figure (a) below:
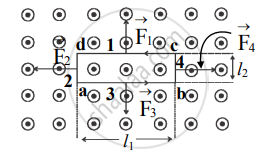
Fig (a): Loop abcd placed in a uniform - The force `vec"F"_4` on side 4 (bc) will be `vec"F"_4` = Il2 B sin(90° - θ)
- The force `vec"F"_2` on side 2 (da) will be equal and opposite to `vec"F"_4` and both act along the same line. Thus, `vec"F"_2` and `vec"F"_4` will cancel out each other.
- The magnitudes of forces `vec"F"_1` and `vec"F"_3` on sides 1 (cd) and 3 (ab) will be Il1 B sin 90° i.e., Il1 B. These two forces do not act along the same line and hence they produce a net torque.
- This torque results into rotation of the loop so that the loop is perpendicular to the direction of `vec"B"`, the magnetic field.
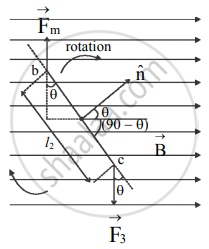
Fig (b): Side view of the loop abcd at an angle θ - Now the moment arm is `1/2 (l_2 sintheta)` about the central axis of the loop. Hence, the torque τ due to forces `vec"F"_1` and `vec"F"_3` will be
τ = `("I l"_1 "B"1/2"l"_2 sintheta) + ("I l"_1 "B"1/2"l"_2 sintheta)`
= `"I" l""_1 "l"_2 "B" sin theta` - If the current-carrying loop is made up of multiple turns N, in the form of a flat coil, the total torque will be
τ' = `"N"tau = "NI""l"_1"l"_2 "B" sintheta`
τ' = (NIA)B sinθ; where A is the are enclosed by the coil = l1l2
This is the required expression.
APPEARS IN
RELATED QUESTIONS
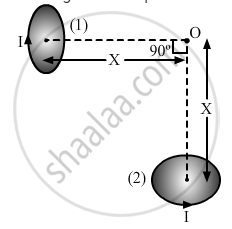
Two very small identical circular loops, (1) and (2), carrying equal currents I are placed vertically (with respect to the plane of the paper) with their geometrical axes perpendicular to each other as shown in the figure. Find the magnitude and direction of the net magnetic field produced at the point O.
Write the expression for the magnetic moment `vecm`due to a planar square loop of side ‘l’ carrying a steady current I in a vector form.
In the given figure this loop is placed in a horizontal plane near a long straight conductor carrying a steady current I1 at a distance l as shown. Give reason to explain that the loop will experience a net force but no torque. Write the expression for this force acting on the loop.

Will a current loop placed in a magnetic field always experience a zero force?
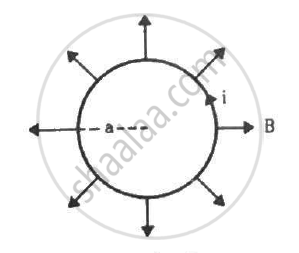
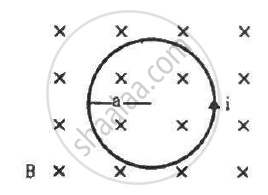
A circular loop of radius a, carrying a current i, is placed in a two-dimensional magnetic field. The centre of the loop coincides with the centre of the field (figure). The strength of the magnetic field at the periphery of the loop is B. Find the magnetic force on the wire.
A rectangular wire-loop of width a is suspended from the insulated pan of a spring balance, as shown in the figure. A current i exists in the anti-clockwise direction in the loop. A magnetic field B exists in the lower region. Find the change in the tension of the spring if the current in the loop is reversed.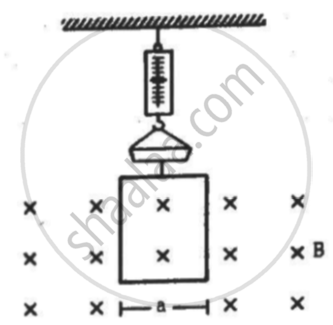
The figure shows a circular wire loop of radius a and carrying a current i, which is placed in a perpendicular magnetic field B. (a) Consider a small part dl of the wire. Find the force on this part of the wire exerted by the magnetic field. (b) Find the force of compression in the wire.
A 50-turn circular coil of radius 2.0 cm carrying a current of 5.0 A is rotated in a magnetic field of strength 0.20 T. (a) What is the maximum torque that acts on the coil? (b) In a particular position of the coil, the torque acting on it is half of this maximum. What is the angle between the magnetic field and the plane of the coil?
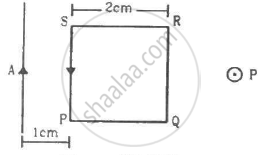
A square loop PQRS carrying a current of 6.0 A is placed near a long wire carrying 10 A as shown in figure. (a) Show that the magnetic force acting on the part PQ is equal and opposite to the part RS. (b) Find the magnetic force on the square loop.
A circular loop of one turn carries a current of 5.00 A. If the magnetic field B at the centre is 0.200 mT, find the radius of the loop.
A current-carrying circular coil of 100 turns and radius 5.0 cm produces a magnetic field of 6.0 × 10−5 T at its centre. Find the value of the current.
Derive the expression for the torque acting on a current-carrying loop placed in a magnetic field.
A moving coil galvanometer has been fitted with a rectangular coil having 50 turns and dimensions 5 cm × 3 cm. The radial magnetic field in which the coil is suspended is of 0.05 Wb/m2. The torsional constant of the spring is 1.5 × 10−9 Nm/degree. Obtain the current required to be passed through the galvanometer so as to produce a deflection of 30°.
A Rectangular coil of 10 turns, each of area 0.05 m2, is suspended freely in a uniform magnetic field of induction 0.01 T. A current of 30 µA is passed through it.
(i) What is the magnetic moment of the coil?
(ii) What is the maximum torque experienced by the coil?
A rectangular coil of length 0.12 m and width 0.1 m having 100 turns of wire is suspended vertically in a uniform magnetic field of strength 0.4 Wb/m2. The coil carries a current of 2.5 A. If the plane of the coil is inclined at an angle of 30° with the direction of the field, the torque required to keep the coil in stable equilibrium will be ____________.
A rectangular coil has 200 turns each of area 50 cm2 . It is capable of rotation about an axis joining the mid points of two opposite sides. When a current of 10 A is passed through it while its plane is at right angles to a uniform magnetic field, it experiences a torque of 5 Nm. The magnetic field will be ____________.
Two galvanometers 'G1' and 'G2' require 2 mA and 3 mA respectively to produce the same deflection. Then _______.
Which one of the following statements is 'NOT' TRUE? Sensitivity of a moving coil galvanometer can be increased by ____________.
In suspended type of moving coil galvanometer ____________.
The magnetic field developed due to current carrying coil at its centre is 'B'. If the new coil of two turns is prepared from the above coil and same current is passed, then the magnetic field at the centre of the new coil will be ____________.
What is the magnetic moment of a current-carrying circular coil if the radius of the circular coil is 'R' and magnetic induction at the center is 'B'?
Two cylinders A and B of the same material have same length, their radii being in the ratio 1 : 2 respectively. The two are joined end to end as shown in the figure. One end of cylinder A is rigidly clamped while free end of cylinder B is twisted through an angle θ. The angle of twist of cylinder A is ______.
When the plane of the coil is parallel to the field, torque will be ______
A current of 10 A is flowing in a wire of length 1.5 m. A force of 15 N acts on it when it is placed in a uniform magnetic field of 2 T. The angle between the magnetic field and the direction of the current is ______.
Calculate the orbital magnetic dipole moment of the electron in the second Bohr orbit of the hydrogen atom, given the radius of the orbit is 2.1 Å and the orbital speed is 1.1 × 106 m/s.
A circular coil having N turns of radius R carrying a current I is used to produce a magnetic field B at its centre O.
If this coil is opened and rewound such that the radius of the newly formed coil is 2R, carrying the same current I, what will be the magnetic field at the centre O?
