Advertisements
Advertisements
Question
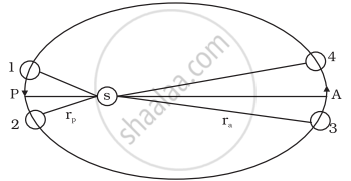
Earth’s orbit is an ellipse with eccentricity 0.0167. Thus, earth’s distance from the sun and speed as it moves around the sun varies from day to day. This means that the length of the solar day is not constant through the year. Assume that earth’s spin axis is normal to its orbital plane and find out the length of the shortest and the longest day. A day should be taken from noon to noon. Does this explain variation of length of the day during the year?
Solution
From the geometry of the ellipse of eccentricity e and semi-major axis a, the aphelion and perihelion distances are:
Angular momentum and areal velocity are constant as the earth orbits the sun
At perigee `r_p^2ω_p = r_a^2ω_a` at apogee.
If 'a' is the semi-major axis of earth's orbit, then `r_p = a(1 - e)` and `r_a = a(l + e)`
∴ `ω_p/ω_a = ((1 + e)/(1 - e))^2, e = 0.0167`
∴ `ω_p/ω_a = 1.0691`
Let ω be angular speed which is the geometric mean of ωp and ωa and corresponds to mean solar day,
∴ `(ω_p/ω) (ω/ω_a) = 1.0691`
∴ `ω_p/ω = ω/ω_a = 1.034`
If ω corresponds to 1° per day (mean angular speed), then w, = 1.034° per day and ωa = 0.967 per day. Since 361° = 14hrs: mean solar day, we get 361.034° which corresponds to 24 hrs 8.14" (8.1" longer) and 360.967° corresponds to 23 hrs 59 min 52" (7.9" smaller).
This does not explain the actual variation in the length of the day during the year.
APPEARS IN
RELATED QUESTIONS
A comet orbits the Sun in a highly elliptical orbit. Does the comet have a constant (a) linear speed, (b) angular speed, (c) angular momentum, (d) kinetic energy, (e) potential energy, (f) total energy throughout its orbit? Neglect any mass loss of the comet when it comes very close to the Sun.
Identify the law shown in the figure and state three respective laws.
Answer the following question.
State Kepler’s law of equal areas.
The square of its period of revolution around the sun is directly proportional to the _______ of the mean distance of a planet from the sun.
The third law of Kepler is also known as the Law of ______.
Supposing Newton’s law of gravitation for gravitation forces F1 and F2 between two masses m1 and m2 at positions r1 and r2 read F1 = – F2 = `- r_12/r_12^3 GM_0^2 ((m_1m_2)/M_0^2)^n` where M0 is a constant of dimension of mass r12 = r1 – r2 and n is a number. in such a case.
- the acceleration due to gravity on earth will be different for different objects.
- none of the three laws of Kepler will be valid.
- only the third law will become invalid.
- for n negative, an object lighter than water will sink in water.
The centre of mass of an extended body on the surface of the earth and its centre of gravity ______.
- are always at the same point for any size of the body.
- are always at the same point only for spherical bodies.
- can never be at the same point.
- is close to each other for objects, say of sizes less than 100 m.
- both can change if the object is taken deep inside the earth.
A star like the sun has several bodies moving around it at different distances. Consider that all of them are moving in circular orbits. Let r be the distance of the body from the centre of the star and let its linear velocity be v, angular velocity ω, kinetic energy K, gravitational potential energy U, total energy E and angular momentum l. As the radius r of the orbit increases, determine which of the above quantities increase and which ones decrease.
lf the angular momentum of a planet of mass m, moving around the Sun in a circular orbit is L, about the center of the Sun, and its areal velocity is ______.
Two planets A and B of equal mass are having their period of revolutions TA and TB such that TA = 2TB. These planets are revolving in the circular orbits of radii rA and rB respectively. Which out of the following would be the correct relationship of their orbits?
