Advertisements
Advertisements
Question
Evaluate the following limits:
`lim_(x -> oo)(1 + 1/x)^(7x)`
Solution
We know `lim_(x -> oo)(x + 1/x)^x` = e
`lim_(x -> oo)(1 + 1/x)^(7x) = [lim_(x -> oo)(1 + 1/x)^x]^7`
= e7
APPEARS IN
RELATED QUESTIONS
Evaluate the following limit:
`lim_(x -> 2)[(x^(-3) - 2^(-3))/(x - 2)]`
Evaluate the following limit :
`lim_(x -> 1)[(x + x^2 + x^3 + ......... + x^"n" - "n")/(x - 1)]`
In the following example, given ∈ > 0, find a δ > 0 such that whenever, |x – a| < δ, we must have |f(x) – l| < ∈.
`lim_(x -> 1) (x^2 + x + 1)` = 3
Evaluate the following :
Find the limit of the function, if it exists, at x = 1
f(x) = `{(7 - 4x, "for", x < 1),(x^2 + 2, "for", x ≥ 1):}`
In problems 1 – 6, using the table estimate the value of the limit
`lim_(x -> 0) (sqrt(x + 3) - sqrt(3))/x`
| x | – 0.1 | – 0.01 | – 0.001 | 0.001 | 0.01 | 0.1 |
| f(x) | 0.2911 | 0.2891 | 0.2886 | 0.2886 | 0.2885 | 0.28631 |
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 5) |x - 5|/(x - 5)`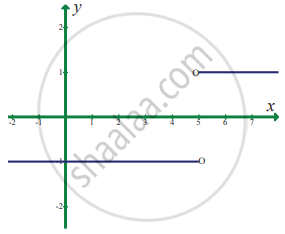
Verify the existence of `lim_(x -> 1) f(x)`, where `f(x) = {{:((|x - 1|)/(x - 1)",", "for" x ≠ 1),(0",", "for" x = 1):}`
Evaluate the following limits:
`lim_(x -> 1) (sqrt(x) - x^2)/(1 - sqrt(x))`
Evaluate the following limits:
`lim_(x -> "a") (sqrt(x - "b") - sqrt("a" - "b"))/(x^2 - "a"^2) ("a" > "b")`
Evaluate the following limits:
`lim_(x -> oo) 3/(x - 2) - (2x + 11)/(x^2 + x - 6)`
Evaluate the following limits:
`lim_(x -> 0) (sin("a" + x) - sin("a" - x))/x`
Evaluate the following limits:
`lim_(x-> 0) (1 - cos x)/x^2`
Evaluate the following limits:
`lim_(x -> 0) (2^x - 3^x)/x`
Choose the correct alternative:
`lim_(x - pi/2) (2x - pi)/cos x`
Choose the correct alternative:
The value of `lim_(x -> 0) sinx/sqrt(x^2)` is
If `lim_(x->1)(x^5-1)/(x-1)=lim_(x->k)(x^4-k^4)/(x^3-k^3),` then k = ______.
`lim_(x -> 5) |x - 5|/(x - 5)` = ______.
`lim_(x -> 0) (sin 4x + sin 2x)/(sin5x - sin3x)` = ______.
`lim_(x→0^+)(int_0^(x^2)(sinsqrt("t"))"dt")/x^3` is equal to ______.
