Advertisements
Advertisements
Question
Figure shows a series LCR circuit connected to a variable frequency 230 V source. L = 5.0 H, C = 80 µF, R = 40 Ω.

- Determine the source frequency which drives the circuit in resonance.
- Obtain the impedance of the circuit and the amplitude of current at the resonating frequency.
- Determine the rms potential drops across the three elements of the circuit. Show that the potential drop across the LC combination is zero at the resonating frequency.
Solution
Inductance of the inductor, L = 5.0 H
Capacitance of the capacitor, C = 80 μF = 80 × 10−6 F
Resistance of the resistor, R = 40 Ω
Potential of the variable voltage source, V = 230 V
(a) Resonance angular frequency is given as:
`ω_"R" = 1/sqrt"LC"`
= `1/sqrt (5 xx 80 xx 10^-6)`
= `10^3/20`
= 50 rad s−1
Hence, the circuit will come in resonance for a source frequency of 50 rad s−1.
(b) Impedance of the circuit is given by the relation,
`"Z" = sqrt("R"^2 + (ω"L" - 1/(ω"C"))^2`
At resonance,
`ω"L" = 1/(ω"C")`
∴ Z = R = 40 Ω
Amplitude of the current at the resonating frequency is given as:
`"I"_0 = "V"_0/"Z"`
Where,
V0 = Peak voltage
= `sqrt2 "V"`
∴ `"I"_0 = (sqrt(2) "V")/"Z"`
= `(sqrt2 xx 230)/4`
= 8.13 A
Hence, at resonance, the impedance of the circuit is 40 Ω and the amplitude of the current is 8.13 A.
(c) The rms potential drop across the inductor,
`("V"_"L")_"rms" = "I" xx ω_"R""L"`
Where,
I = rms current
= `"I"_0/sqrt2`
= `(sqrt2 "V")/(sqrt2 "Z")`
= `230/40 "A"`
∴ `("V"_"L")_"rms"= 230/40 xx 50 xx 5`
= 1437.5 V
Potential drop across the capacitor,
`("V"_"c")_"rms" = "I" xx 1/(ω_"R" "C")`
= `230/40 xx 1/(50 xx 80 xx 10^-6)`
= 1437.5 V
Potential drop across the resistor,
`("V"_"R")_"rms" = "IR"`
= `230/40 xx 40`
= 230 V
Potential drop across the LC combination,
`"V"_"LC" = "I" (ω_"R" "L" - 1/(ω_"R" "C"))`
At resonance, ωRL = `1/(ω_"R""C")`
∴ VLC = 0
Hence, it is proved that the potential drop across the LC combination is zero at resonating frequency.
APPEARS IN
RELATED QUESTIONS
Define 'quality factor' of resonance in a series LCR circuit. What is its SI unit?
In a series LCR circuit, VL = VC ≠ VR. What is the value of power factor?
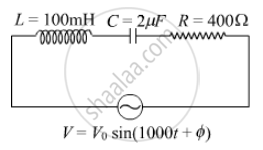
(i) Find the value of the phase difference between the current and the voltage in the series LCR circuit shown below. Which one leads in phase : current or voltage ?
(ii) Without making any other change, find the value of the additional capacitor C1, to be connected in parallel with the capacitor C, in order to make the power factor of the circuit unity.
In a series LCR circuit, obtain the condition under which watt-less current flows in the circuit ?
An inductor-coil of inductance 17 mH is constructed from a copper wire of length 100 m and cross-sectional area 1 mm2. Calculate the time constant of the circuit if this inductor is joined across an ideal battery. The resistivity of copper = 1.7 × 10−8 Ω-m.
A coil having an inductance L and a resistance R is connected to a battery of emf ε. Find the time taken for the magnetic energy stored in the circuit to change from one fourth of the steady-state value to half of the steady-state value.
An inductor of inductance 2.00 H is joined in series with a resistor of resistance 200 Ω and a battery of emf 2.00 V. At t = 10 ms, find (a) the current in the circuit, (b) the power delivered by the battery, (c) the power dissipated in heating the resistor and (d) the rate at which energy is being stored in magnetic field.
Draw a labelled graph showing a variation of impedance of a series LCR circuit with frequency of the a.c. supply.
Answer the following question.
In a series LCR circuit connected across an ac source of variable frequency, obtain the expression for its impedance and draw a plot showing its variation with frequency of the ac source.
A series LCR circuit with R = 20 Ω, L = 1.5 H and C = 35 µF is connected to a variable-frequency 200 V ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transferred to the circuit in one complete cycle?
Obtain the resonant frequency and Q-factor of a series LCR circuit with L = 3.0 H, C = 27 µF, and R = 7.4 Ω. It is desired to improve the sharpness of the resonance of the circuit by reducing its ‘full width at half maximum’ by a factor of 2. Suggest a suitable way.
For a series LCR-circuit, the power loss at resonance is ______.
Assertion: When the frequency of the AC source in an LCR circuit equals the resonant frequency, the reactance of the circuit is zero, and so there is no current through the inductor or the capacitor.
Reason: The net current in the inductor and capacitor is zero.
At resonance frequency the impedance in series LCR circuit is ______.
A series LCR circuit containing 5.0 H inductor, 80 µF capacitor and 40 Ω resistor is connected to 230 V variable frequency ac source. The angular frequencies of the source at which power transferred to the circuit is half the power at the resonant angular frequency are likely to be ______.
If the rms current in a 50 Hz ac circuit is 5 A, the value of the current 1/300 seconds after its value becomes zero is ______.
As the frequency of an ac circuit increases, the current first increases and then decreases. What combination of circuit elements is most likely to comprise the circuit?
- Inductor and capacitor.
- Resistor and inductor.
- Resistor and capacitor.
- Resistor, inductor and capacitor.
When an alternating voltage of 220V is applied across device X, a current of 0.25A flows which lags behind the applied voltage in phase by π/2 radian. If the same voltage is applied across another device Y, the same current flows but now it is in phase with the applied voltage.
- Name the devices X and Y.
- Calculate the current flowing in the circuit when the same voltage is applied across the series combination of X and Y.
Draw a labelled graph showing variation of impedance (Z) of a series LCR circuit Vs frequency (f) of the ac supply. Mark the resonant frequency as f0·
