Advertisements
Advertisements
Question
Find the coordinates of O, the centre passing through A( -2, -3), B(-1, 0) and C(7, 6). Also, find its radius.
Solution
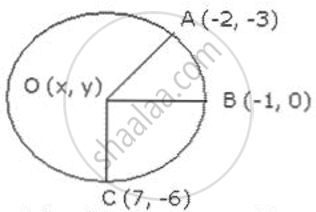
let o (x, y) be the centre of the circle.
OA = OB (radii of square circle)
⇒ OA2 = OB2
(x + 2)2 + (y + 3)2 = (x + 1)2 + (y - 0)2
⇒ x2 + 4 + 4x + y2 + 9 + 6y = x2 + 1 + 2x + y2
⇒ 2x + 6y + 12 = 0
⇒ x + 3y + 6 = 0 .........(1)
Similarly, OB=OC
⇒ OB2 =OC2
(x + 1)2 + (y + 0)2 = (x + 7)2 + (y - 6)2
⇒ x2 + 1 + 2x + y2 = x2 + 49 - 14x + y2 + 36 +12y
⇒ 16x - 12y - 84 = 0
⇒ 4x - 3y - 21 = 0 .... (2)
⇒ 4x + 12y +24 = 0 .....(1)
on solving (1)& (2) we get,
- 15 y - 45 = 0
⇒ y = -3
from (1)
x - 9 + 6 = 0
⇒ x = 3
Thus coordinate of O are (3 , -3)
Radius =`sqrt ((3 + 2)^2 + (- 3 + 3)^2)`
`= sqrt (25 + 0)`
` = sqrt 25` units
= 5 units
APPEARS IN
RELATED QUESTIONS
If the opposite vertices of a square are (1, – 1) and (3, 4), find the coordinates of the remaining angular points.
If A (-1, 3), B (1, -1) and C (5, 1) are the vertices of a triangle ABC, find the length of the median through A.
Find the value of m if the distance between the points (m , -4) and (3 , 2) is 3`sqrt 5` units.
A(-2, -3), B(-1, 0) and C(7, -6) are the vertices of a triangle. Find the circumcentre and the circumradius of the triangle.
Prove that the points (6 , -1) , (5 , 8) and (1 , 3) are the vertices of an isosceles triangle.
Find distance between point A(–1, 1) and point B(5, –7):
Solution: Suppose A(x1, y1) and B(x2, y2)
x1 = –1, y1 = 1 and x2 = 5, y2 = – 7
Using distance formula,
d(A, B) = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2`
∴ d(A, B) = `sqrt(square +[(-7) + square]^2`
∴ d(A, B) = `sqrt(square)`
∴ d(A, B) = `square`
Show that the points (2, 0), (– 2, 0) and (0, 2) are vertices of a triangle. State the type of triangle with reason
The equation of the perpendicular bisector of line segment joining points A(4,5) and B(-2,3) is ______.
The points (– 4, 0), (4, 0), (0, 3) are the vertices of a ______.
The point A(2, 7) lies on the perpendicular bisector of line segment joining the points P(6, 5) and Q(0, – 4).
