Advertisements
Advertisements
Question
Prove that the points (6 , -1) , (5 , 8) and (1 , 3) are the vertices of an isosceles triangle.
Solution
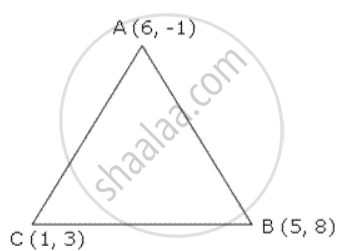
AB = `sqrt ((6 - 5)^2 + (-1-8)^2) = sqrt (1 + 81) = sqrt 82` units
BC = `sqrt ((5-1)^2 + (8 - 3)^2) = sqrt (16+25) = sqrt 41` units
AC = `sqrt ((1-6)^2 + (3 - 1)^2) = sqrt (25 + 16) = sqrt 41` units
∵ BC = AC
∴ A , B and C are the vertices of an isosceles triangle.
APPEARS IN
RELATED QUESTIONS
Find the distance of a point (12 , 5) from another point on the line x = 0 whose ordinate is 9.
Find the distance between P and Q if P lies on the y - axis and has an ordinate 5 while Q lies on the x - axis and has an abscissa 12 .
A point A is at a distance of `sqrt(10)` unit from the point (4, 3). Find the co-ordinates of point A, if its ordinate is twice its abscissa.
What point on the x-axis is equidistant from the points (7, 6) and (-3, 4)?
Calculate the distance between A (7, 3) and B on the x-axis, whose abscissa is 11.
The distance between points P(–1, 1) and Q(5, –7) is ______
Point P(0, 2) is the point of intersection of y-axis and perpendicular bisector of line segment joining the points A(–1, 1) and B(3, 3).
The points A(–1, –2), B(4, 3), C(2, 5) and D(–3, 0) in that order form a rectangle.
Find distance between points P(– 5, – 7) and Q(0, 3).
By distance formula,
PQ = `sqrt(square + (y_2 - y_1)^2`
= `sqrt(square + square)`
= `sqrt(square + square)`
= `sqrt(square + square)`
= `sqrt(125)`
= `5sqrt(5)`
The distance of the point (5, 0) from the origin is ______.
