Advertisements
Advertisements
Question
Find the area of the following triangle:

Solution
Area of triangle = `1/2 xx "Base" xx "Height"`
Base = 5 cm,
Height = 3.2 cm
Area = `1/2 xx 5 xx 3.2`
= 8 cm2
APPEARS IN
RELATED QUESTIONS
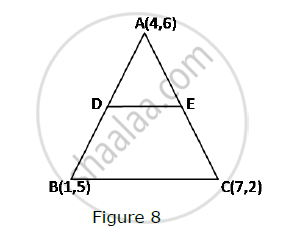
In Fig. 8, the vertices of ΔABC are A(4, 6), B(1, 5) and C(7, 2). A line-segment DE is drawn to intersect the sides AB and AC at D and E respectively such that `(AD)/(AB)=(AE)/(AC)=1/3 `Calculate th area of ADE and compare it with area of ΔABCe.
In each of the following find the value of 'k', for which the points are collinear.
(8, 1), (k, -4), (2, -5)
Find equation of line joining (1, 2) and (3, 6) using the determinant.
ΔABC is right angled at A (see the given figure). AD is perpendicular to BC. If AB = 5 cm, BC = 13 cm and AC = 12 cm, Find the area of ΔABC. Also find the length of AD.

The point A divides the join of P (−5, 1) and Q(3, 5) in the ratio k:1. Find the two values of k for which the area of ΔABC where B is (1, 5) and C(7, −2) is equal to 2 units.
Prove analytically that the line segment joining the middle points of two sides of a triangle is equal to half of the third side.
Find the value of y for which the points A(-3, 9), B(2,y) and C(4,-5) are collinear.
Find the value(s) of k so that the quadratic equation x2 − 4kx + k = 0 has equal roots.
Points A(3, 1), B(12, –2) and C(0, 2) cannot be the vertices of a triangle.
Area of a triangle = `1/2` base × ______.
