Advertisements
Advertisements
Question
Find two positive numbers x and y such that x + y = 60 and xy3 is maximum.
Solution
Let the two number be x and y and x + y = 60. ...(i)
Let P = xy3
⇒ P = (60 - y)y3 ...[from(i)]
⇒ P = 60y3 - y4
⇒ `(dP)/dy = 180y^2 - 4y^3`
For maximum P, we must have `(dP)/dy = 0`
⇒ 180y2 - 4y3 = 0
⇒ 4y2 (45 - y) = 0
⇒ y = 45 ...(∵ 0 < y < 60)
Also, `(d^2P)/dy^2 = 360y - 12y^2 and`
`((d^2P)/dy^2)_(y = 45) = 360 xx 45 - 12 xx (45)^2 < 0`
Therefore, P is maximum when y = 45
∴ The required numbers are x = 60 - y = 60 - 45 = 15 and y = 45.
APPEARS IN
RELATED QUESTIONS
Examine the maxima and minima of the function f(x) = 2x3 - 21x2 + 36x - 20 . Also, find the maximum and minimum values of f(x).
Find the approximate value of cos (89°, 30'). [Given is: 1° = 0.0175°C]
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
`h(x) = sinx + cosx, 0 < x < pi/2`
Find both the maximum value and the minimum value of 3x4 − 8x3 + 12x2 − 48x + 25 on the interval [0, 3].
Show that of all the rectangles inscribed in a given fixed circle, the square has the maximum area.
Show that the right circular cone of least curved surface and given volume has an altitude equal to `sqrt2` time the radius of the base.
A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is 10 m. Find the dimensions of the window to admit maximum light through the whole opening
A given quantity of metal is to be cast into a half cylinder with a rectangular base and semicircular ends. Show that in order that the total surface area may be minimum the ratio of the length of the cylinder to the diameter of its semi-circular ends is \[\pi : (\pi + 2)\].
Find the point on the straight line 2x+3y = 6, which is closest to the origin.
Find the maximum and minimum of the following functions : f(x) = `x^2 + (16)/x^2`
Solve the following:
Find the maximum and minimum values of the function f(x) = cos2x + sinx.
The function f(x) = x log x is minimum at x = ______.
A wire of length 120 cm is bent in the form of a rectangle. Find its dimensions if the area of the rectangle is maximum
A rectangular sheet of paper has it area 24 sq. Meters. The margin at the top and the bottom are 75 cm each and the sides 50 cm each. What are the dimensions of the paper if the area of the printed space is maximum?
A rod of 108 m long is bent to form a rectangle. Find it’s dimensions when it’s area is maximum.
The minimum value of the function f(x) = 13 - 14x + 9x2 is ______
The sum of two non-zero numbers is 6. The minimum value of the sum of their reciprocals is ______.
Let f have second derivative at c such that f′(c) = 0 and f"(c) > 0, then c is a point of ______.
If the sum of the surface areas of cube and a sphere is constant, what is the ratio of an edge of the cube to the diameter of the sphere, when the sum of their volumes is minimum?
If x is real, the minimum value of x2 – 8x + 17 is ______.
The smallest value of the polynomial x3 – 18x2 + 96x in [0, 9] is ______.
The curves y = 4x2 + 2x – 8 and y = x3 – x + 13 touch each other at the point ______.
Find the volume of the largest cylinder that can be inscribed in a sphere of radius r cm.
The function `"f"("x") = "x" + 4/"x"` has ____________.
The combined resistance R of two resistors R1 and R2 (R1, R2 > 0) is given by `1/"R" = 1/"R"_1 + 1/"R"_2`. If R1 + R2 = C (a constant), then maximum resistance R is obtained if ____________.
Let f(x) = 1 + 2x2 + 22x4 + …… + 210x20. Then f (x) has ____________.
The function `f(x) = x^3 - 6x^2 + 9x + 25` has
A function f(x) is maximum at x = a when f'(a) > 0.
If p(x) be a polynomial of degree three that has a local maximum value 8 at x = 1 and a local minimum value 4 at x = 2; then p(0) is equal to ______.
If the point (1, 3) serves as the point of inflection of the curve y = ax3 + bx2 then the value of 'a ' and 'b' are ______.
Let x and y be real numbers satisfying the equation x2 – 4x + y2 + 3 = 0. If the maximum and minimum values of x2 + y2 are a and b respectively. Then the numerical value of a – b is ______.
The maximum value of z = 6x + 8y subject to constraints 2x + y ≤ 30, x + 2y ≤ 24 and x ≥ 0, y ≥ 0 is ______.
If f(x) = `1/(4x^2 + 2x + 1); x ∈ R`, then find the maximum value of f(x).
If Mr. Rane order x chairs at the price p = (2x2 - 12x - 192) per chair. How many chairs should he order so that the cost of deal is minimum?
Solution: Let Mr. Rane order x chairs.
Then the total price of x chairs = p·x = (2x2 - 12x- 192)x
= 2x3 - 12x2 - 192x
Let f(x) = 2x3 - 12x2 - 192x
∴ f'(x) = `square` and f''(x) = `square`
f'(x ) = 0 gives x = `square` and f''(8) = `square` > 0
∴ f is minimum when x = 8
Hence, Mr. Rane should order 8 chairs for minimum cost of deal.
Find the maximum and the minimum values of the function f(x) = x2ex.
Find the local maxima and local minima, if any, of the following function. Find also the local maximum and the local minimum values, as the case may be:
f(x) `= x sqrt(1 - x), 0 < x < 1`
Sumit has bought a closed cylindrical dustbin. The radius of the dustbin is ‘r' cm and height is 'h’ cm. It has a volume of 20π cm3.
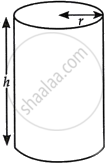
- Express ‘h’ in terms of ‘r’, using the given volume.
- Prove that the total surface area of the dustbin is `2πr^2 + (40π)/r`
- Sumit wants to paint the dustbin. The cost of painting the base and top of the dustbin is ₹ 2 per cm2 and the cost of painting the curved side is ₹ 25 per cm2. Find the total cost in terms of ‘r’, for painting the outer surface of the dustbin including the base and top.
- Calculate the minimum cost for painting the dustbin.
