Advertisements
Advertisements
Question
From the rate expression for the following reaction, determine the order of reaction and the dimension of the rate constant.
\[\ce{H2O2_{( aq)} + 3I^-_{( aq)} + 2H^+ -> 2H2O_{(l)} + I^-_3}\] Rate = k[H2O2][I−]
Solution
Given rate = k[H2O2][I−]
Therefore, order of the reaction = 2
Dimension of k = `"Rate"/(["H"_2"O"_2]["I"^(-)])`
= `("mol L"^(-1) "s"^(-1))/(("mol L"^(-1))("mol L"^(-1)))`
= L mol−1 s−1
APPEARS IN
RELATED QUESTIONS
Define “zero order reaction”.
In a first order reaction x → y, 40% of the given sample of compound remains unreacted in 45 minutes. Calculate rate constant of the reaction.
For the first order thermal decomposition reaction, the following data were obtained:
Time / sec Totalpressure / atm
0 0.30
300 0.50
Calculate the rate constant
(Given: log 2 = 0.301, log3 = 0.4771, log 4 = 0.6021)
For the hydrolysis of methyl acetate in aqueous solution, the following results were obtained :
| t/s | 0 | 30 | 60 |
| [CH3COOCH3] / mol L–1 | 0.60 | 0.30 | 0.15 |
(i) Show that it follows pseudo first order reaction, as the concentration of water remains constant.
(ii) Calculate the average rate of reaction between the time interval 30 to 60 seconds.
(Given log 2 = 0.3010, log 4 = 0.6021)
For the reaction: \[\ce{2A + B → A2B}\] the rate = k[A][B]2 with k = 2.0 × 10−6 mol−2 L2 s−1. Calculate the initial rate of the reaction when [A] = 0.1 mol L−1, [B] = 0.2 mol L−1. Calculate the rate of reaction after [A] is reduced to 0.06 mol L−1.
A reaction is second order with respect to a reactant. How is the rate of reaction affected if the concentration of the reactant is doubled?
Define the following terms:
Half-life period of reaction (t1/2).
Consider a first order gas phase decomposition reaction given below :
\[\ce{A(g) -> B(g) + C(g)}\]
The initial pressure of the system before decomposition of A was pi. After lapse of time ‘t’, total pressure of the system increased by x units and became ‘pt’ The rate constant k for the reaction is given as ______.
Which of the following statements is not correct about order of a reaction.
Consider the reaction A ⇌ B. The concentration of both the reactants and the products varies exponentially with time. Which of the following figures correctly describes the change in concentration of reactants and products with time?
Why can we not determine the order of a reaction by taking into consideration the balanced chemical equation?
Match the graph given in Column I with the order of reaction given in Column II. More than one item in Column I may link to the same item of Column II.
| Column I | Column II | |
| (i) | 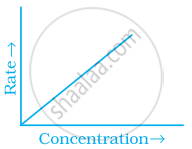 |
|
| (ii) |  |
(a) 1st order |
| (iii) | 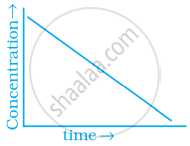 |
(b) Zero-order |
| (iv) | 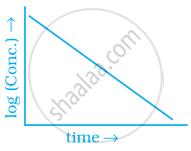 |
Assertion: Order of the reaction can be zero or fractional.
Reason: We cannot determine order from balanced chemical equation.
Assertion: Rate constants determined from Arrhenius equation are fairly accurate for simple as well as complex molecules.
Reason: Reactant molecules undergo chemical change irrespective of their orientation during collision.
The role of a catalyst is to change
In the presence of a catalyst, the heat evolved or absorbed during the reaction.
For a reaction 1/2 A ⇒ 2B, rate of disappearance of A is related 't o the appearance of B by the expression:
The half-life period of a. substance in a certain enzyme catalysed reaction is 138 s. The time required for the concentration of the substance to fall from 1.28 mol–1 to 0.04 mg L–1 is
The conversion of molecules A to B follow second order kinetics. If concentration of A is increased to three times, how will it affect the rate of formation of B?
