Advertisements
Advertisements
Question
If an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram.
Solution
\[\text{ Two adjacent angles of a parallelogram add up to } 180° . \]
\[\text{ Let x be the angle } . \]
\[ \therefore x + \frac{2x}{3} = 180°\]
\[\frac{5x}{3} = = 180°\]
\[x = 72°\]
\[\frac{2x}{3} = \frac{2 \times 72°}{3} = 108°\]
\[\text{Thus, two of the angles in the parallelogram are 108° and the other two are }72° .\]
APPEARS IN
RELATED QUESTIONS
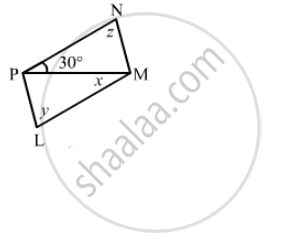
The following figure is parallelogram. Find the degree values of the unknown x, y, z.
In the following figure GUNS and RUNS are parallelogram. Find x and y.

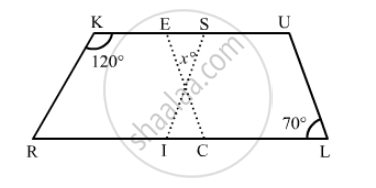
In the following figure RISK and CLUE are parallelograms. Find the measure of x.
Two adjacent angles of a parallelogram are as 1 : 2. Find the measures of all the angles of the parallelogram.
In a parallelogram ABCD, ∠D = 135°, determine the measure of ∠A and ∠B.
Find the angles marked with a question mark shown in Fig. 17.27

In the following Figure ABCD is a arallelogram, CE bisects ∠C and AF bisects ∠A. In each of the following, if the statement is true, give a reason for the same:
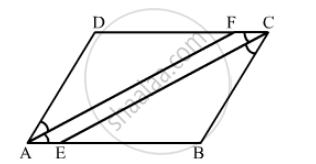
(i) ∠A = ∠C
(ii) \[\angle FAB = \frac{1}{2}\angle A\]
(iii) \[\angle DCE = \frac{1}{2}\angle C\]
(iv) \[\angle CEB = \angle FAB\]
(v) CE || AF
Fill in the blank, in the following, so as to make the statement true:
The diagonals of a rhombus ...... each other at ...... angles.
ABCD is a rhombus whose diagonals intersect at O. If AB = 10 cm, diagonal BD = 16 cm, find the length of diagonal AC.
In rhombus ABCD;
(i) if ∠A = 74° ; find ∠B and ∠C.
(ii) if AD = 7.5 cm ; find BC and CD.
