Advertisements
Advertisements
Question
If from an external point P of a circle with centre O, two tangents PQ and PR are drawn such that ∠QPR = 120°, prove that 2PQ = PO.
Solution
Let us draw the circle with external point P and two tangents PQ and PR.

We know that the radius is perpendicular to the tangent at the point of contact.
∴∠OQP=90°
We also know that the tangents drawn to a circle from an external point are equally inclined to the segment, joining the centre to that point.
∴∠QPO=60°
Now in ∆QPO:
`cos60^@="PQ"/"PO"`
`⇒1/2="PQ"/"PO"`
`⇒2PQ=PO`
APPEARS IN
RELATED QUESTIONS
From an external point P, tangents PA and PB are drawn to a circle with centre O. If ∠PAB = 50°, then find ∠AOB.
In the given figure, XY and X’Y’ are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XY at A and X’Y’ at B. Prove that ∠AOB = 90°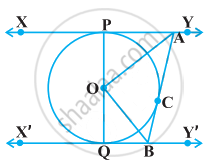
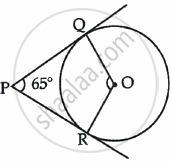
In the figure given below, O is the center of the circle and SP is a tangent. If ∠SRT = 65°, find the value of x, y and Z.

In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.

Find: ∠AOB
Construct a pair of tangents to a circle of radius 4 cm from a point which is at a distance of 6 cm from its centre.
In fig, O is the centre of the circle, CA is tangent at A and CB is tangent at B drawn to the circle. If ∠ACB = 75°, then ∠AOB = ______

The angle between two tangents to a circle may be 0°.
If angle between two tangents drawn from a point P to a circle of radius a and centre O is 90°, then OP = `asqrt(2)`.
In figure, common tangents AB and CD to two circles intersect at E. Prove that AB = CD.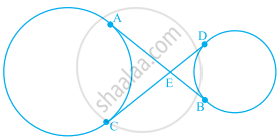
In the given figure, PQ and PR are tangents drawn from P to the circle with centre O such that ∠QPR = 65°. The measure of ∠QOR is ______.