Advertisements
Advertisements
Question
If tan x° = `(5)/(12) . tan y° = (3)/(4)` and AB = 48m; find the length CD.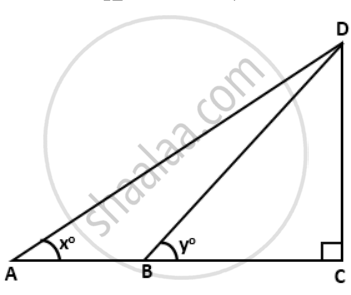
Solution

tan x = `"CD"/"AC"`
⇒ `(5)/(12) = "CD"/"AC"`
⇒ 5 x AC = 12 x CD
⇒ 5(AB + BC) = 12CD
⇒ 5(48 + BC) = 12CD
⇒ 48 + BC = `(12"CD")/(5)` ....(i)
tan y = `"CD"/"BC"`
⇒ `(3)/(4) = "CD"/"BC"`
⇒ 3BC = 4CD
⇒ BC = `(6"CD")/(3)` ....(ii)
Substituting (ii) in (i), we have
`48 + (4"CD")/(3) = (12"CD")/(5)`
⇒ `(12"CD")/(5) - (4"CD")/(3)` = 48
⇒ `(36"CD" - 20"CD")/(15)` = 48
⇒ 16CD = 720
⇒ CD = 45m.
APPEARS IN
RELATED QUESTIONS
Solve the following equation for A, if tan 3 A = 1
Solve for x : sin (x + 10°) = `(1)/(2)`
If A = B = 60°, verify that: cos(A - B) = cosA cosB + sinA sinB
In a right triangle ABC, right angled at C, if ∠B = 60° and AB = 15units, find the remaining angles and sides.
Find the value of 'y' if `sqrt(3)` = 1.723.
Given your answer correct to 2 decimal places.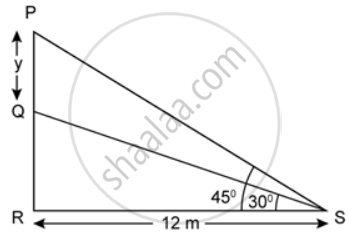
Evaluate the following: `(sec32° cot26°)/(tan64° "cosec"58°)`
Evaluate the following: sin35° sin45° sec55° sec45°
If sec2θ = cosec3θ, find the value of θ if it is known that both 2θ and 3θ are acute angles.
If A, B and C are interior angles of ΔABC, prove that sin`(("A" + "B")/2) = cos "C"/(2)`
If secθ= cosec30° and θ is an acute angle, find the value of 4 sin2θ - 2 cos2θ.
