Advertisements
Advertisements
Question
PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T. Find the lengths of TP and TQ.

Solution

Given,
Radius, OP = 5 cm
PQ = 8 cm,
TP = TQ (Tangents drawn from a common point to a circle are equal)
Δ PTQ is isosceles.
Let PQ and OT intersect at point M.
TO is the angle bisector of ∠PTQ.
So, ∠PMT = 90° ⇒ PM = QM = 4 cm .... Perpendicular drawn from the center of the circle to the chord bisects the chord)
Given,
Radius, OP = 5 cm
PQ = 8 cm,
TP = TQ (Tangents drawn from a common point to a circle are equal)
Δ PTQ is isosceles.
Let PQ and OT intersect at point M.
TO is the angle bisector of ∠PTQ.
So, ∠PMT = 90° ⇒ PM = QM = 4 cm .... Perpendicular drawn from the center of the circle to the chord bisects the chord)
In Δ PMO,
OP2 = OM2 + PM2
⇒ 52 = OM2 + 42
⇒ OM = 3 cm
Let PT = x and TM = y
In Δ PMT,
PT2 = TM2 + PM2
x2 = y2 + 16 .....(i)
In Δ TPO,
OT2 = PT2 + OP2
(y + 3)2 = x2 + 52
y2 + 9 + 6y = x2 + 25 ....(ii)
From (i) and (ii), we get
y2 + 9 + 6y = y2 + 16 + 25
6y = 32
y = `16/3` cm
substituting y = `16/3`in equation (i),
`"x"^2 = (16/3)^2 + 16`
`x^2 = 400/9`
`x = 20/3` cm
Therefore, PT = `20/3` cm
RELATED QUESTIONS
A chord of a circle of radius 10 em subtends a right angle at its centre. The length of the chord (in em) is
`(A) 5sqrt 2`
`(B) 10 sqrt2`
`(C)5/sqrt2`
`(D) 10sqrt 3`
ABC is a right triangle, right angled at B. A circle is inscribed in it. The lengths of the two sides containing the right angle are 6 cm and 8 cm. Find the radius of the incircle.
O is the center of a circle of radius 8cm. The tangent at a point A on the circle cuts a line through O at B such that AB = 15 cm. Find OB
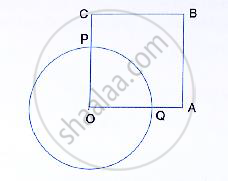
In the following figure, OABC is a square. A circle is drawn with O as centre which meets OC
at P and OA at Q. Prove that:
(i) ΔOPA ≅ ΔOQC, (ii) ΔBPC ≅ ΔBQA.
A chord of length 14 cm is at a distance of 6 cm from the centre of a circle. The length of another chord at a distance of 2 cm from the centre of the circle is
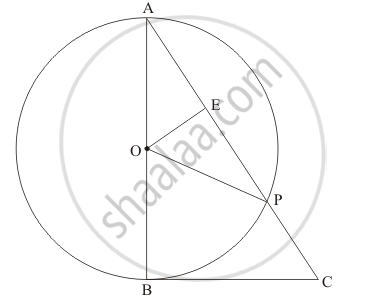
In the given figure, BC is a tangent to the circle with centre O. OE bisects AP. Prove that ΔAEO ∼ Δ ABC.
Use the figure given below to fill in the blank:
Diameter = 2 x ________

State, if the following statement is true or false:
The diameters of a circle always pass through the same point in the circle.
AD is a diameter of a circle and AB is a chord. If AD = 34 cm, AB = 30 cm, the distance of AB from the centre of the circle is ______.
From the figure, identify a diameter.

