Advertisements
Advertisements
Question
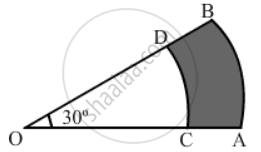
In the following figure, the shaded area is

Options
50 (π−2) cm2
25 (π−2) cm2
25 (π+2) cm2
5 (π−2) cm2
Solution
In the figure,∠AOB = 90°
and radius of the circle = 10 cm
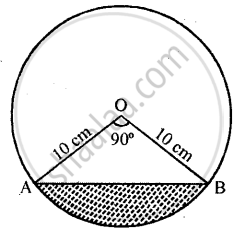
∴ Area of shaded portion = Area of quadrant AOB - area of ΔAOB
= `1/4pi"r"^2-1/2"r"^2`
= `1/4"r"^2(pi-2)`cm2
= `1/4xx10xx10(pi-2)`cm2
= 25 (π - 2) cm2
APPEARS IN
RELATED QUESTIONS
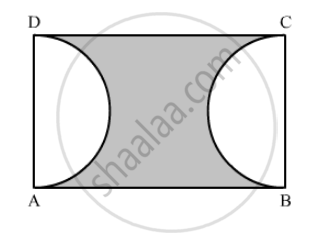
In the following figure, ABCD is a rectangle, having AB = 20 cm and BC = 14 cm. Two sectors of 180° have been cut off. Calculate:
the area of the shaded region.
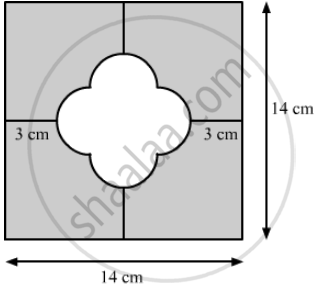
In the following figure find the area of the shaded region. (Use π = 3.14)
A circular park has a path of uniform width around it. The difference between the outer and inner circumferences of the circular path is 132 m. Its width is
If π is taken as `22/7` the distance (in metres) covered by a wheel of diameter 35 cm, in one revolution, is
ABCD is a rectangle whose three vertices are B (4,0), C (4,3) and D (0, 3). The length of one of its diagonals is
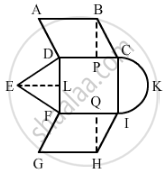
A child draws the figure of an aeroplane as shown. Here, the wings ABCD and FGHI are parallelograms, the tail DEF is an isosceles triangle, the cockpit CKI is a semicircle and CDFI is a square. In the given figure, BP ⊥ CD, HQ ⊥ FI and EL ⊥ DF. If CD = 8 cm, BP = HQ = 4 cm and DE = EF = 5 cm, find the area of the whole figure.
In the given figure, the sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area of the shaded region.
Find the radius and area of a circle, whose circumference is :
(i) 132 cm
(ii) 22 m
The diameters of three wheels are in the ratio 2 : 4 : 8. If the sum of the circumferences of these circles be 132 cm, find the difference between the areas of the largest and the smallest of these wheels.
Area of a quadrant of a circle of radius 7 cm is ______.
