Advertisements
Advertisements
प्रश्न
In the following figure, the shaded area is

पर्याय
50 (π−2) cm2
25 (π−2) cm2
25 (π+2) cm2
5 (π−2) cm2
उत्तर
In the figure,∠AOB = 90°
and radius of the circle = 10 cm
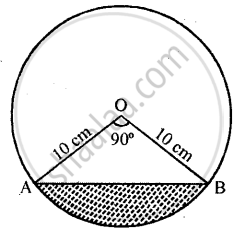
∴ Area of shaded portion = Area of quadrant AOB - area of ΔAOB
= `1/4pi"r"^2-1/2"r"^2`
= `1/4"r"^2(pi-2)`cm2
= `1/4xx10xx10(pi-2)`cm2
= 25 (π - 2) cm2
APPEARS IN
संबंधित प्रश्न
In Figure 5, a circle is inscribed in a triangle PQR with PQ = 10 cm, QR = 8 cm and PR =12 cm. Find the lengths of QM, RN and PL ?

ABCDEF is a regular hexagon with centre O (in the following figure). If the area of triangle OAB is 9 cm2, find the area of : (i) the hexagon and (ii) the circle in which the haxagon is incribed.
A wire can be bent in the form of a circle of radius 56 cm. If it is bent in the form fo a square, then its area will be
If the area of a sector of a circle bounded by an arc of length 5π cm is equal to 20π cm2, then its radius is
The area of the circle that can be inscribed in a square of side 10 cm is
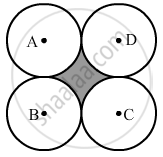
Four equal circles, each of radius 5 cm, touch each other, as shown in the figure. Find the area included between them.
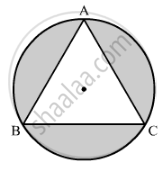
On a circular table cover of radius 42 cm, a design is formed by a girl leaving an equilateral triangle ABC in the middle, as shown in the figure. Find the covered area of the design. `["Use" sqrt(3) = 1.73, pi =22/7]`
Perimeter of a sector of a circle whose central angle is 90° and radius 7 cm is ____________.
In figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
Read the following passage:
People of a circular village Dharamkot want to construct a road nearest to it. The road cannot pass through the village. But the people want the road at a shortest distance from the centre of the village. Suppose the road starts from A which is outside the circular village (as shown in the figure) and touch the boundary of the circular village at B such that AB = 20 m. Also the distance of the point A from the centre O of the village is 25 m. |
Based on the above information, answer the following questions:
- If B is the mid-point of AC, then find the distance AC.
- Find the shortest distance of the road from the centre of the village.
- Find the circumference of the village.
OR
Find the area of the village.


