Advertisements
Advertisements
Question
In given fig., quadrilateral PQRS, side PQ || side SR, AR = 5 AP, then prove that, SR = 5PQ
Solution
side PQ || side SR and seg SQ is their transversal. ...[Given]
∴ ∠QSR ≅ ∠SQP ...[Alternative angles]
∴ ∠ASR ≅ ∠AQP ......(i) [Q−A−S]
In ΔASR and ΔAQP,
∠ASR ≅ ∠AQP ......[From (i)]
∠SAR ≅ ∠QAP ......[Vertically opposite angles]
∴ ΔASR ∼ ΔAQP ......[AA test of similarity]
∴ `"AR"/"AP" = "SR"/"PQ"` ......(ii)[Corresponding sides of similar triangles]
But, AR = 5 AP ......[Given]
∴ `"AR"/"AP" = 5/1` ......(iii)
∴ `"SR"/"PQ" = 5/1` ......[From (ii) and (iii)]
∴ SR = 5PQ
RELATED QUESTIONS
The diagonals of a quadrilateral ABCD intersect each other at the point O such that `("AO")/("BO") = ("CO")/("DO")`. Show that ABCD is a trapezium.
In the given figure, ∠CAB = 90° and AD⊥BC. Show that ΔBDA ~ ΔBAC. If AC = 75cm, AB = 1m and BC = 1.25m, find AD.

ABCD is parallelogram and E is a point on BC. If the diagonal BD intersects AE at F, prove that AF × FB = EF × FD.

In the given figure, ∠1 = ∠2 and `(AC)/(BD)=(CB)/(CE)` Prove that Δ ACB ~ Δ DCE.
ΔABC~ΔDEF and their areas are respectively 64 cm2 and 121cm2. If EF = 15.4cm, find BC.
In ∆ABC, ray BD bisects ∠ABC and ray CE bisects ∠ACB. If seg AB ≅ seg AC then prove that ED || BC.
The actual area of an island is 1872km2 . On a map, this area is 117 cm2. if the length of the coastline is 44cm on the map, find the length of its actual coastline.
A triangle ABC has been enlarged by scale factor m = 2.5 to the triangle A' B' C'. Calculate : the length of AB, if A' B' = 6 cm.
A triangle LMN has been reduced by scale factor 0.8 to the triangle L' M' N'. Calculate: the length of LM, if L' M' = 5.4 cm.
Sides of a triangle are 7, 24 and 25. Determine whether the triangle is a right-angled triangle or not.
D and E are points on the sides AB and AC respectively of a Δ ABC such that DE | | BC and divides Δ ABC into two parts, equal in area. Find `"BD"/"AB"`.
In figure, PQ is parallel to BC, AP : AB = 2 : 7. If QC = 0 and BC = 21,
Find
(i) AQ
(ii) PQ
In ΔABC, point D divides AB in the ratio 5:7, Find: BC, If DE = 2.5cm
In ΔABC, point D divides AB in the ratio 5:7, Find: DE, If BC = 4.8cm
ΔABC is enlarged, with a scale factor 5. Find: A'B', if AB = 4cm
D is the mid point of side BC and AE ⊥ BC. If BC = a, AC = b, AB = c, ED = x, AD = p and AE = h, prove that b2 = `"p"^2 + "a"x + "a"^2/4`
In the given figure YH || TE. Prove that ΔWHY ~ ΔWET and also find HE and TE
Are triangles in figure similar? If yes, then write the test of similarity.
Prove that if a line is drawn parallel to one side of a triangle intersecting the other two sides in distinct points, then the other two sides are divided in the same ratio.
Using the above theorem prove that a line through the point of intersection of the diagonals and parallel to the base of the trapezium divides the non-parallel sides in the same ratio.
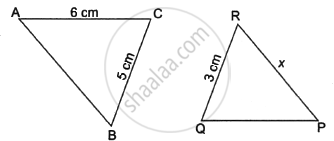
In the given figure, ΔABC ∼ ΔQPR, If AC = 6 cm, BC = 5 cm, QR = 3 cm and PR = x; them the value of x is ______.