Advertisements
Advertisements
Question
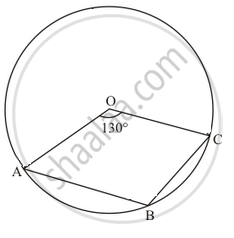
In the given figure, O is the centre of the circle such that ∠AOC = 130°, then ∠ABC =
Options
130°
115°
65°
165°
Solution
115°
We have the following information in the following figure. Take a point P on the circle in the given figure and join AP and CP.

Since, the angle subtended by a chord at the centre is twice that of subtended atany part of the circle.
So, `angleAPC = (angleAOC)/2 = 130/2 = 65°`
Since `squareAPBP` is a cyclic quadrilateral and we known that opposite angles are supplementary.
Therefore,
\[ \Rightarrow \angle ABC + 65° = 180° \]
\[ \Rightarrow \angle ABC = 180° - 65° \]
\[ \Rightarrow \angle ABC = 115°\]
APPEARS IN
RELATED QUESTIONS
Prove that "Opposite angles of a cyclic quadrilateral are supplementary".
A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
ABCD is a cyclic quadrilateral in ∠BCD = 100° and ∠ABD = 70° find ∠ADB.
If the two sides of a pair of opposite sides of a cyclic quadrilateral are equal, prove that its diagonals are equal.
If a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral so formed is cyclic.
If non-parallel sides of a trapezium are equal, prove that it is cyclic.
If P, Q and R are the mid-points of the sides BC, CA and AB of a triangle and AD is the perpendicular from A on BC, prove that P, Q, R and D are concyclic.
The three angles of a quadrilateral are 100°, 60°, 70°. Find the fourth angle.
