Advertisements
Advertisements
Question
If P, Q and R are the mid-points of the sides BC, CA and AB of a triangle and AD is the perpendicular from A on BC, prove that P, Q, R and D are concyclic.
Advertisements
Solution
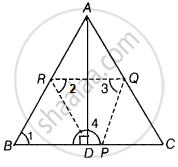
Given: In ΔABC, P, Q and R are the mid-points of the sides BC, CA and AB respectively. Also, AD ⊥ BC.
To prove: P, Q, R and D are concyclic.
Construction: Join DR, RQ and QP
Proof: In right-angled ΔADP, R is the mid-point of AB.
∴ RB = RD
⇒ ∠2 = ∠1 ...(i) [Angles opposite to the equal sides are equal]
Since, R and Q are the mid-points of AB and AC, then
RQ || BC ...[By mid-point theorem]
or RQ || BP
Since, QP || RB, then quadrilateral BPQR is a parallelogram.
⇒ ∠1 = ∠3 ...(ii) [Opposite angles of parallelogram are equal]
From equations (i) and (ii),
∠2 = ∠3
But ∠2 + ∠4 = 180° ...[Linear pair axiom]
∴ ∠3 + ∠4 = 180° ...[∴ ∠2 = ∠3]
Hence, quadrilateral PQRD is a cyclic quadrilateral.
So, points P, Q, R and D are non-cyclic.
Hence proved.
APPEARS IN
RELATED QUESTIONS
A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠DBC = 70°, ∠BAC is 30°, find ∠BCD. Further, if AB = BC, find ∠ECD.
Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively (see the given figure). Prove that ∠ACP = ∠QCD.
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.
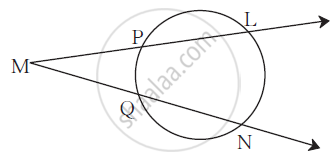
In the figure m(arc LN) = 110°,
m(arc PQ) = 50° then complete the following activity to find ∠LMN.
∠ LMN = `1/2` [m(arc LN) - _______]
∴ ∠ LMN = `1/2` [_________ - 50°]
∴ ∠ LMN = `1/2` × _________
∴ ∠ LMN = __________
Circles are described on the sides of a triangle as diameters. Prove that the circles on any two sides intersect each other on the third side (or third side produced).
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140º, then ∠BAC is equal to ______.
If a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral so formed is cyclic.
In the following figure, AOB is a diameter of the circle and C, D, E are any three points on the semi-circle. Find the value of ∠ACD + ∠BED.
