Advertisements
Advertisements
Question
In the given figure O is the centre of the circle. Tangents A and B meet at C. If ∠ACO = 30°, find
1) ∠BCO
2) ∠AOB
3) ∠APB
Solution
In ΔAOC, ACO = 30° (Given)

∠OAC = 90° [radius is perpendicular to the tangent at the point of contact]
By angle sum property, ACO + OAC + AOC = 180o
AOC = 180° – (90° + 30°) = 60°
Consider Δ AOC and Δ BOC
AO = BO (radii)
AC = BC (tangents to a circle from an external point are equal in length)
OC = OC (Common)
ΔAOC ≅ ΔBOC
1) ∠BCO = ∠ACO = 30°
2) ∠AOC = ∠BOC = 60°
∠AOB = ∠AOC + ∠BOC = 120°
3) We know that, “If two angles stand on the same chord, then the angle at the centre is twice the angle at the circumference.
∠AOB and ∠APB stand on the same chord AB.
∠AOB = 2∠APB
So ∠APB = 1/2 ∠AOB = 60°
APPEARS IN
RELATED QUESTIONS
Draw a circle of radius 3.5 cm. Marks a point P outside the circle at a distance of 6 cm from the centre. Construct two tangents from P to the given circle. Measure and write down the length of one tangent.
The given figure shows a circle with centre O and BCD is tangent to it at C. Show that : ∠ACD + ∠BAC = 90°.

In the given figure AC is a tangent to the circle with centre O.
If ∠ADB = 55° , find x and y. Give reasons for your answers.

Find the length of the tangent from a point which is at a distance of 5cm from the centre of the circle of radius 3cm.
In following fig., ABC is a right- angled triangle at A with sides AB = 5 cm and BC = 13 cm . A circle with centre O has been inscribed in the triangle ABC. Calculate the radius of the incircle.
In followinf fig., two concentric circles with centre 0 are of radii 5 cm and 3 cm. from an external point P, tangents PA and PB are drawn to these circles. If AP = 12cm, find BP.

In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If angle ACO = 30°, find: angle APB

Find the area of sector whose central angle and radius are 60o and 21 cm respectively.
`(pi = 22/7)`
The quadrilateral formed by joining the angle bisectors of a cyclic quadrilateral is a ______
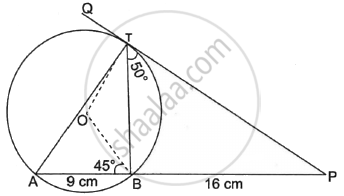
In the given figure, O is the centre of the circle. PQ is a tangent to the circle at T. Chord AB produced meets the tangent at P.
AB = 9 cm, BP = 16 cm, ∠PTB = 50° ∠OBA = 45°
Find:
- Length of PT
- ∠BAT
- ∠BOT
- ∠ABT