Advertisements
Advertisements
Question
In the adjoining figure, PQ is the diameter, chord SR is parallel to PQ. Give ∠ PQR = 58°.
Calculate:
(i) ∠ RPQ,
(ii) ∠ STP ( T is a point on the minor arc)
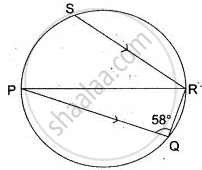
Solution
(i) ∠ PRQ = 90° ...(∠in a semicircle)
In Δ PQR,
∠ RPQ + ∠ PQR + ∠ PRQ = 180° ....(∵ The sum of the three ∠s of a Δ is 180°)
⇒ ∠ RPQ + 58° + 90° = 180°
⇒ ∠ RPQ + 148° = 180°
⇒ ∠ RPQ = 180° - 148°
⇒ ∠ RPQ = 32°
(ii) ∵ PQ || SR and RP intersects them
∠ PRS = ∠ RPQ ...(Alternate angles)
∴ ∠ PRS = 32°
∵ PTSR is a cyclic quadrilateral.
∴ ∠ PTS + ∠ PRS = 180° ....( ∵ Opposite ∠s of a cyclic quadrilateral are supplementary)
⇒ ∠ PTS + 32° = 180°
⇒ ∠ PTS = 180° - 32° = 148°
⇒ ∠ STP = 148°.
APPEARS IN
RELATED QUESTIONS
In the following figure, O is the centre of the circle. Find the values of a, b, c and d.
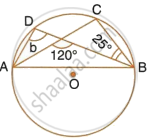
In the following figure, O is the centre of the circle. Find the values of a, b, c and d

In the following figure, O is the centre of the circle. Find the values of a, b, c and d.
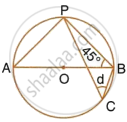
Calculate:
- ∠CDB,
- ∠ABC,
- ∠ACB.
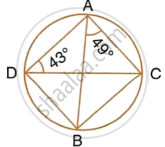
Given: ∠CAB = 75° and ∠CBA = 50°. Find the value of ∠DAB + ∠ABD.

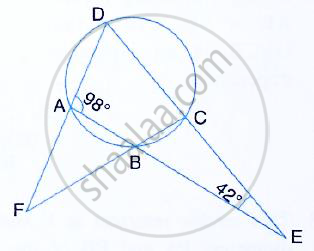
The sides AB and DC of a cyclic quadrilateral ABCD are produced to meet at E; the sides DA and CB are produced to meet at F. If ∠BEC = 42° and ∠BAD = 98°; Calculate :
(i) ∠AFB (ii) ∠ADC
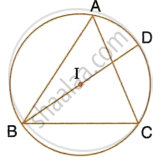
In the given figure, I is the incentre of ΔABC. BI when produced meets the circumcircle of ΔABC at D. ∠BAC = 55° and ∠ACB = 65°; calculate:
- ∠DCA,
- ∠DAC,
- ∠DCI,
- ∠AIC.
In following fig., chords PQ and RS of a circle intersect at T. If RS = 18cm, ST = 6cm and PT = 18cm, find the length of TQ.

The length of the common chord of two intersecting circles is 30 cm. If the diameters of these two circles are 50 cm and 34 cm, calculate the distance between their centers.
If a diameter of a circle bisects each of the two chords of a circle, prove that the chords are parallel.
