Advertisements
Advertisements
Question
In the figure, AB and CD are common tangents to two circles of unequal radii. Prove that AB = CD.
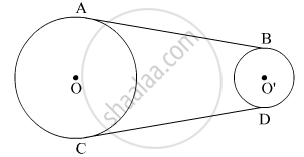
Solution 1
Construct a line passing through AD
Now, AD and CD are tangents to the circle with centre O from the external point D.
So, AD = CD (Tangents drawn from an external point to a circle are equal) ...(1)
Also, AB and AD are the tangents to the circle with centre O' from the external point A.
So, AD = AB (Tangents drawn from an external point to a circle are equal) ...(2)
From (1) and (2)
AB = CD
Hence proved.
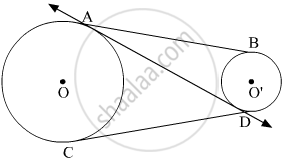
Solution 2
Given: AB and CD are two common tangents to two circles of unequal radii.
To Prove: AB = CD
Construction: Produce AB and CD, to intersect at P.
Proof: Consider the circle with greater radius.
AP = CP ...[Tangents drawn from an external point to a circle are equal] [1]
Also, Consider the circle with smaller radius.
BP = BD ...[Tangents drawn from an external point to a circle are equal] [2]
Substract [2] from [1], we get
AP – BP = CP – BD
AB = CD
Hence proved.
RELATED QUESTIONS
From an external point P, tangents PA and PB are drawn to a circle with centre O. If ∠PAB = 50°, then find ∠AOB.
Prove that the tangents drawn at the end points of a chord of a circle make equal angles with the chord.
Two concentric circles of radii a and b (a > b) are given. Find the length of the chord of the larger circle which touches the smaller circle.
Construct a pair of tangents to a circle of radius 4 cm from a point which is at a distance of 6 cm from its centre.
The length of the tangent from an external point on a circle is ______
In a circle of radius 5 cm, AB and AC are the two chords such that AB = AC = 6 cm. Find the length of the chord BC.
The angle between two tangents to a circle may be 0°.
In the given figure, O is the centre of circle. Find ∠AQB, given that PA and PB are tangents to the circle and ∠APB = 75°.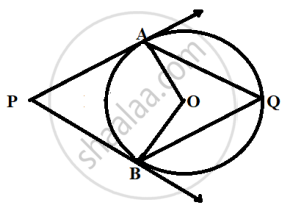
In the given figure, PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q meet at a point T. Find the length of TP.

From an external point, two tangents are drawn to a circle. Prove that the line joining the external point to the centre of the circle bisects the angle between the two tangents.
