Advertisements
Advertisements
Question
In the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that: BD = CD

Solution
In ΔADB and ΔADC,
∠ADB = ∠ADC ...(Since AD is perpendicular to BC)
AB = AC ...(given)
AD = AD ...(common side)
∴ ΔADB ≅ ΔADC ...(RHS congruence criterion)
⇒ BD = CD ...(cpct)
APPEARS IN
RELATED QUESTIONS
You want to show that ΔART ≅ ΔPEN,
If you have to use SSS criterion, then you need to show
1) AR =
2) RT =
3) AT =

In Fig. 10.99, AD ⊥ CD and CB ⊥. CD. If AQ = BP and DP = CQ, prove that ∠DAQ = ∠CBP.

Which of the following statements are true (T) and which are false (F):
Two right triangles are congruent if hypotenuse and a side of one triangle are respectively equal equal to the hypotenuse and a side of the other triangle.
In the given figure, prove that:
CD + DA + AB + BC > 2AC
If the following pair of the triangle is congruent? state the condition of congruency :
In Δ ABC and Δ DEF, AB = DE, BC = EF and ∠ B = ∠ E.
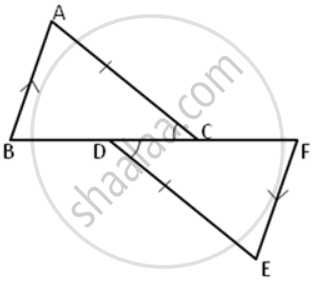
Use the information in the given figure to prove:
- AB = FE
- BD = CF
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD. Prove that:
AB = CE.
In the following figure, BL = CM.

Prove that AD is a median of triangle ABC.
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
Which of the following is not a criterion for congruence of triangles?
