Advertisements
Advertisements
Question
In the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that : ED = EF

Solution
In ΔEFB and ΔEDB,
∠EFB = ∠EDB ( both are 900 )
EB = EB ( common side )
∠FBE = ∠DBE ( given )
ΔEFB ≅ ΔEDB (AAS congruence criterion)
⇒ EF = ED (cpct )
that is , Ed = EF.
APPEARS IN
RELATED QUESTIONS
In the given figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.

If ΔABC and ΔPQR are to be congruent, name one additional pair of corresponding parts. What criterion did you use?
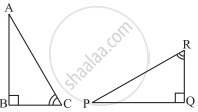
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
In the given figure, prove that:
CD + DA + AB > BC
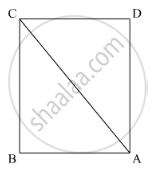
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
If the following pair of the triangle is congruent? state the condition of congruency:
In ΔABC and ΔPQR, AB = PQ, AC = PR, and BC = QR.
The given figure shows a circle with center O. P is mid-point of chord AB.

Show that OP is perpendicular to AB.
In the following diagram, ABCD is a square and APB is an equilateral triangle.
(i) Prove that: ΔAPD ≅ ΔBPC
(ii) Find the angles of ΔDPC.
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
In a triangle, ABC, AB = BC, AD is perpendicular to side BC and CE is perpendicular to side AB.
Prove that: AD = CE.
