Advertisements
Advertisements
Question
In the given figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.

Solution
Given that
∠BAD = ∠EAC
On adding ∠DAC on both sides, we get
∠BAD + ∠DAC = ∠EAC + ∠DAC
⇒ ∠BAC = ∠EAD …(I)
Now, in △ABC and △AED,
AB = AD ...[Given]
AC = AE ...[Given]
∠BAC = ∠EAD ...[By (I)]
∴ △ABC ≌ △ADE ...[By AAS congruence rule]
⇒ BC = DE ...[Corresponding parts of congruent triangles]
APPEARS IN
RELATED QUESTIONS
AD and BC are equal perpendiculars to a line segment AB (See the given figure). Show that CD bisects AB.

In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the given figure). Show that:
- ΔAMC ≅ ΔBMD
- ∠DBC is a right angle.
- ΔDBC ≅ ΔACB
- CM = `1/2` AB

You want to show that ΔART ≅ ΔPEN,
If it is given that AT = PN and you are to use ASA criterion, you need to have
1) ?
2) ?

In ΔABC, ∠A = 30°, ∠B = 40° and ∠C = 110°
In ΔPQR, ∠P = 30°, ∠Q = 40° and ∠R = 110°
A student says that ΔABC ≅ ΔPQR by AAA congruence criterion. Is he justified? Why or why not?
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
The following figure shows a circle with center O.
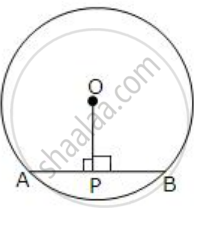
If OP is perpendicular to AB, prove that AP = BP.
In the given figure, AB = DB and Ac = DC.

If ∠ ABD = 58o,
∠ DBC = (2x - 4)o,
∠ ACB = y + 15o and
∠ DCB = 63o ; find the values of x and y.
In the following figure, BL = CM.

Prove that AD is a median of triangle ABC.
In the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the triangle PQR.
If XS ⊥ QR and XT ⊥ PQ ;
prove that: (i) ΔXTQ ≅ ΔXSQ.
(ii) PX bisects angle P.
ABC is a right triangle with AB = AC. Bisector of ∠A meets BC at D. Prove that BC = 2AD.
