Advertisements
Advertisements
Question
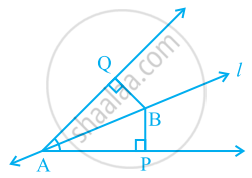
Line l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A (see the given figure). Show that:
- ΔAPB ≅ ΔAQB
- BP = BQ or B is equidistant from the arms of ∠A.
Solution
We have, l is the bisector of ∠QAP.
∴ ∠QAB = ∠PAB
and ∠Q = ∠P ...[Each 90°]
⇒ ∠ABQ = ∠ABP ...[By angle sum property of △]
i. Now, in △APB and △AQB, we have
∠ABP = ∠ABQ ...[Proved above]
AB = BA ...[Common]
∠PAB ≅ ∠QAB ...[Given]
△APB ≅ △AQB ...[By ASA congruency]
ii. Since, △APB ≅ △AQB
⇒ BP = BQ ...[By Corresponding parts of congruent triangles]
i.e., [Perpendicular distance of B from AP] = [Perpendicular distance of B from AQ]
Thus, the point B is equidistant from the arms of ∠A.
APPEARS IN
RELATED QUESTIONS
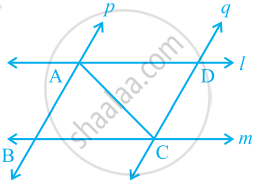
l and m are two parallel lines intersected by another pair of parallel lines p and q (see the given figure). Show that ΔABC ≅ ΔCDA.
In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the given figure). Show that:
- ΔAMC ≅ ΔBMD
- ∠DBC is a right angle.
- ΔDBC ≅ ΔACB
- CM = `1/2` AB

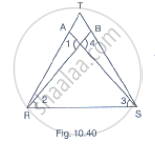
In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT.
In Δ ABC, ∠B = 35°, ∠C = 65° and the bisector of ∠BAC meets BC in P. Arrange AP, BP and CP in descending order.
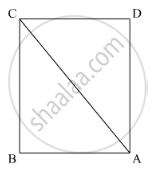
In the given figure, prove that:
CD + DA + AB + BC > 2AC
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
The given figure shows a circle with center O. P is mid-point of chord AB.

Show that OP is perpendicular to AB.
A triangle ABC has ∠B = ∠C.
Prove that: The perpendiculars from the mid-point of BC to AB and AC are equal.
In the following figure, BL = CM.

Prove that AD is a median of triangle ABC.
AD and BC are equal perpendiculars to a line segment AB. If AD and BC are on different sides of AB prove that CD bisects AB.
