Advertisements
Advertisements
Question
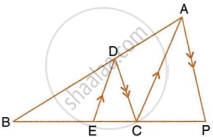
In the following figure, AB, CD and EF are perpendicular to the straight line BDF.
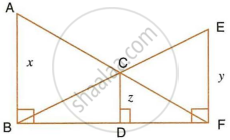
If AB = x and CD = z unit and EF = y unit, prove that : `1/x + 1/y = 1/z`
Solution
In ΔFDC and ΔFBA,
∠FDC = ∠FBA ...(Since DC || AB)
∠DFC = ∠BFA ...(Common angle)
ΔFDC ∼ ΔFBA ...(AA criterion for similarity)
`=> (DC)/(AB) = (DF)/(BF)`
`=> z/x = (DF)/(BF)` ...(i)
In ΔBDC and ΔBFE,
∠BDC = ∠BFE ...(Since DC || FE)
∠DBC = ∠FBE ...(Common angle)
ΔBDC ∼ ΔBFE ...(AA criterion for similarity)
`=> (BD)/(BF) = (DC)/(EF)`
`=> (BD)/(BF) = z/y` ...(ii)
Adding (i) and (ii), we get
`(BD)/(BF) + (DF)/(BF) = z/y + z/x`
`=> 1 = z/y + z/x`
`=> 1/z = 1/x + 1/y`
Hence proved.
APPEARS IN
RELATED QUESTIONS
State, true or false:
Two isosceles triangles are similar, if an angle of one is congruent to the corresponding angle of the other.
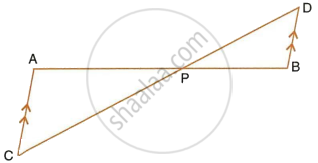
In the figure, given below, straight lines AB and CD intersect at P; and AC || BD. Prove that: If BD = 2.4 cm, AC = 3.6 cm, PD = 4.0 cm and PB = 3.2 cm; find the lengths of PA and PC.
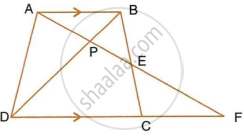
In the following figure, ABCD to a trapezium with AB || DC. If AB = 9 cm, DC = 18 cm, CF = 13.5 cm, AP = 6 cm and BE = 15 cm, Calculate: AF
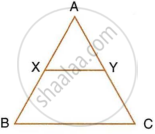
In the following figure, ∠AXY = ∠AYX. If `(BX)/(AX) = (CY)/(AY)`, show that triangle ABC is isosceles.
In the following figure, DE || AC and DC || AP. Prove that : `(BE)/(EC) = (BC)/(CP)`.
Triangles ABC and DEF are similar.
If area (ΔABC) = 16 cm, area (ΔDEF) = 25 cm2 and BC = 2.3 cm find EF.
Triangles ABC and DEF are similar.
If area (ΔABC) = 36 cm2, area (ΔDEF) = 64 cm2 and DE = 6.2 cm, find AB.
In the adjoining figure ABC is a right angle triangle with ∠BAC = 90°, and AD ⊥ BC.
(i) Prove ΔADB ∼ ΔCDA.
(ii) If BD = 18 cm, CD = 8 cm find AD.
(iii) Find the ratio of the area of ΔADB is to area of ΔCDA.
Triangles ABC and DEF are similar.
If AC = 19 cm and DF = 8 cm, find the ratio between the areas of two triangles.
Triangles ABC and DEF are similar.
If area (ΔABC) = 36 cm2, area (ΔDEf) = 64 cm2 and DE = 6.2 cm, find AB.
