Advertisements
Advertisements
Question
In the given circle with diameter AB, find the value of x.
Solution
∠ ABD = ∠ ACD = 30° ....(∠s of same segment)
∠ADB = 90° ....(∠ in the semi circle)
In ΔADB,
x° + 90° + 30° = 180° ....(Sum of all ∠s of triangle)
x° = 180° - 120°
x° = 60°.
APPEARS IN
RELATED QUESTIONS
ABCD is a quadrilateral such that ∠D = 90°. A circle (O, r) touches the sides AB, BC, CD and DA at P,Q,R and If BC = 38 cm, CD = 25 cm and BP = 27 cm, find r.
A circle is inscribed in a ΔABC touching AB, BC and AC at P, Q and R respectively. If AB = 10 cm, AR=7cm and CR=5cm, find the length of BC.

In the given figure, O is the centre of the circle and ∠BDC = 42°. The measure of ∠ACB is

Find the area of a circle of radius 7 cm.
Use the figure given below to fill in the blank:
If PQ is 8 cm long, the length of RS = ________

A point A is 26 cm away from the centre of a circle and the length of the tangent drawn from A to the circle is 24 cm. Find the radius of the circle.

Two circles with centres O and O' of radii 3 cm and 4 cm, respectively intersect at two points P and Q such that OP and O'P are tangents to the two circles. Find the length of the common chord PQ.
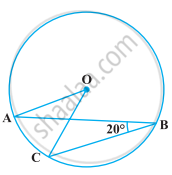
In the following figure, if ∠ABC = 20º, then ∠AOC is equal to ______.
Draw two acute angles and one obtuse angle without using a protractor. Estimate the measures of the angles. Measure them with the help of a protractor and see how much accurate is your estimate
Say true or false:
The centre of a circle is always in its interior.
