Advertisements
Advertisements
Question
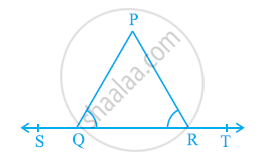
In the given figure, ∠PQR = ∠PRQ, then prove that ∠PQS = ∠PRT.
Solution
Given: ∠PQR = ∠PRQ
To prove: ∠PQS = ∠PRT
Proof:
∠PQS + ∠PQR = 180° ...(1) Linear pair
∠PRT + ∠PRQ = 180° ...(2) Linear pair
From equations (1) and (2)
∠PQS + ∠PQR = ∠PRT + ∠PRQ
or, ∠PQS + ∠PQR = ∠PRT + ∠PQR ...(∠PQR = ∠PRQ is given)
or, ∠PQS + ∠PQR = ∠PRT + ∠PQR
or ∠PQS = ∠PRT is proved.
APPEARS IN
RELATED QUESTIONS
Write the complement of the following angles .
30°
If the angles (2x −10)° and (x − 5)° are complementary angles, find x.
An angle is 14° more than its complementary angle. What is its measure?
The measure of an angle is twice the measure of its supplementary ang Find its measure.
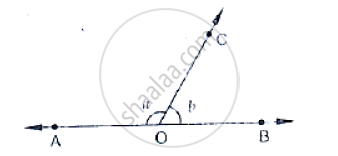
In the below Fig, ∠AOC and ∠BOC form a linear pair. if a − 2b = 30°, find a and b.
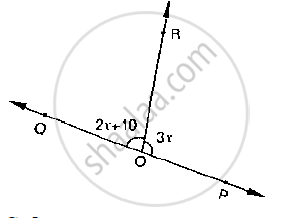
Given ∠POR = 3x and ∠QOR = 2x + 10, find the value of x for which POQ will be a line.
(Below fig).
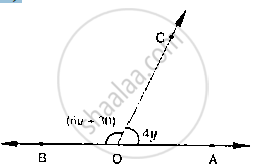
What value of y would make AOB a line in below fig, if ∠AOC = 4y and ∠BOC = (6y +
30)
If below fig, ∠AOF and ∠FOG form a linear pair.
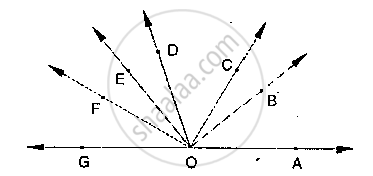
∠EOB = ∠FOC = 90° and ∠DOC = ∠FOG = ∠AOB = 30°
(i) Find the measures of ∠FOE, ∠COB and ∠DOE.
(ii) Name all the right angles.
(iii) Name three pairs of adjacent complementary angles.
(iv) Name three pairs of adjacent supplementary angles.
(v) Name three pairs of adjacent angles.
statement are true and false
Angles forming a linear pair are supplementary.
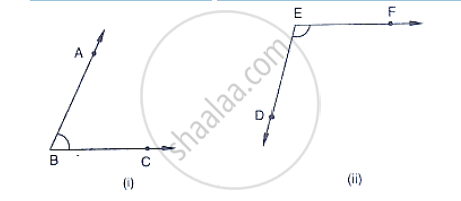
In the below fig, arms BA and BC of ∠ABC are respectively parallel to arms ED and EF of
∠DEF. Prove that ∠ABC + ∠DEF = 180°.