Advertisements
Advertisements
Question
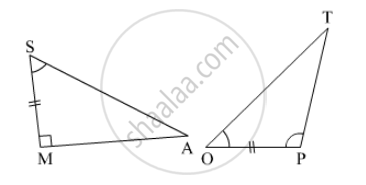
In the pair of triangles in the following figure, parts bearing identical marks are congruent. State the test and the correspondence of vertices by the triangle in pairs is congruent.
Solution
ΔSMA and ΔOPT
∠S ≅ ∠O ....[corresponding angles are equal]
∠A ≅ ∠T ....[corresponding angles are equal]
SM ≅ OP ....[corresponding sides are equal]
Here, the two triangles are congruent by the ASA test, in the correspondence SMA ↔ OPT.
RELATED QUESTIONS
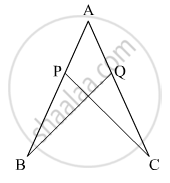
In the given figure, if AB = AC and ∠B = ∠C. Prove that BQ = CP.
ΔPQR and ΔABC is not congruent to ΔRPQ, then which of the following is not true:
In an isosceles triangle, if the vertex angle is twice the sum of the base angles, then the measure of vertex angle of the triangle is
Which of the following is not a criterion for congruence of triangles?
In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangle in each pair are congruent.

By ______ test
ΔXYZ ≅ ΔLMN
The following figure has shown a triangle ABC in which AB = AC. M is a point on AB and N is a point on AC such that BM = CN.
Prove that: (i) BN = CM (ii) ΔBMC≅ΔCNB

State, whether the pairs of triangles given in the following figures are congruent or not:

In the figure, AB = EF, BC = DE, AB and FE are perpendiculars on BE. Prove that ΔABD ≅ ΔFEC
In ΔABC, AD is a median. The perpendiculars from B and C meet the line AD produced at X and Y. Prove that BX = CY.
It is given that ∆ABC ≅ ∆RPQ. Is it true to say that BC = QR? Why?
